Lecture
Among the few flat figures, the probability of hitting which can be calculated in the final form, belongs to the ellipse of dispersion (an ellipse of equal density).
Let the normal law on the plane be given in canonical form:
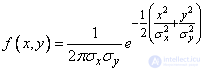 . (9.4.1)
. (9.4.1)
Consider the scattering ellipse 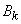 whose equation
whose equation
 ,
,
where is the parameter 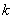 is the ratio of the semiaxes of the dispersion ellipse to the main standard quadratic deviations. According to the general formula (8.3.3) we have:
is the ratio of the semiaxes of the dispersion ellipse to the main standard quadratic deviations. According to the general formula (8.3.3) we have:
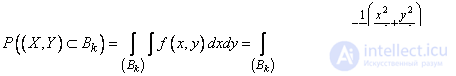 . (9.4.2)
. (9.4.2)
We make in the integral (9.4.2) the change of variables
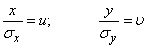 .
.
This substitution ellipse 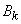 converted to a circle
converted to a circle  radius
radius  . Consequently,
. Consequently,
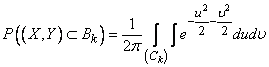 . (9.4.3)
. (9.4.3)
We proceed in the integral (9.4.3) from the Cartesian coordinate system to the polar one, setting
 . (9.4.4)
. (9.4.4)
The conversion Jacobian (9.4.4) is 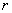 . Making the change of variables, we get:
. Making the change of variables, we get:
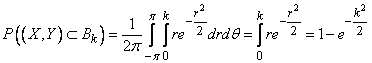 .
.
Thus, the probability of hitting a random point in the dispersion ellipse, whose semi-axes are equal 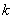 standard deviations equal to:
standard deviations equal to:
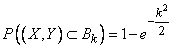 . (9.4.5)
. (9.4.5)
As an example, we find the probability of hitting a random point distributed according to the normal law on the plane 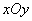 in the unit ellipse of dispersion, whose semi-axes are equal to the mean square deviations:
in the unit ellipse of dispersion, whose semi-axes are equal to the mean square deviations:
 .
.
For such an ellipse 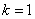 . We have:
. We have:
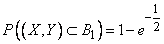
Using table 2 of the application, we find:
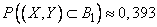 .
.
Formula (9.4.5) is most often used to calculate the probability of hitting a circle with circular scattering.
Example. On the path of a fast moving small size target  put a fragmentation field in the form of a flat disk of radius
put a fragmentation field in the form of a flat disk of radius 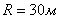 . Inside the disk, the density of the fragments is constant and equal to
. Inside the disk, the density of the fragments is constant and equal to 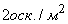 . If the target is covered with a disk, then the number of fragments falling into it can be considered distributed according to the Poisson law. Due to the smallness of the target, it can be considered as a point and it can be considered that it is either completely covered by a fragmentation field (if its center falls within a fragmentation circle), or not at all covered (if its center does not fall within a circle). A hit of a shard guarantees defeat of the target. When aiming center circle
. If the target is covered with a disk, then the number of fragments falling into it can be considered distributed according to the Poisson law. Due to the smallness of the target, it can be considered as a point and it can be considered that it is either completely covered by a fragmentation field (if its center falls within a fragmentation circle), or not at all covered (if its center does not fall within a circle). A hit of a shard guarantees defeat of the target. When aiming center circle 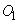 seek to combine in the plane
seek to combine in the plane 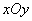 with origin
with origin 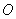 (target center), but due to point errors
(target center), but due to point errors 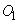 scatters about
scatters about 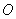 (fig. 9.4.1). The law of dispersion is normal, the dispersion is circular,
(fig. 9.4.1). The law of dispersion is normal, the dispersion is circular, 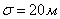 . Determine the probability of hitting the target.
. Determine the probability of hitting the target. 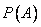 .
.
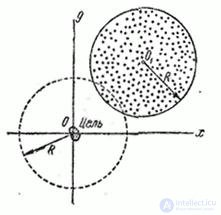
Fig. 9.4.1
Decision. For a target to be hit by shrapnel, it is necessary to combine two events: 1) hitting a target (point 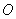 ) in a fragmentation field (circle of radius
) in a fragmentation field (circle of radius 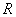 ) and 2) defeat the target, provided that the hit occurred.
) and 2) defeat the target, provided that the hit occurred.
The probability of hitting a target in a circle is obviously equal to the probability that the center of the circle (a random point 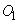 ) gets into a circle of radius
) gets into a circle of radius 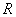 , described around the origin. Apply the formula (9.4.5). We have:
, described around the origin. Apply the formula (9.4.5). We have:
 .
.
The probability of hitting a target in a fragmentation field is:
 .
.
Next, we find the probability of hitting the target. 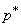 provided it is covered with a fragmentation disk. Average number of shards
provided it is covered with a fragmentation disk. Average number of shards  that fall into a covered field target is equal to the product of the target area and the density of the fragment field:
that fall into a covered field target is equal to the product of the target area and the density of the fragment field:
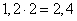 .
.
Conditional probability of hitting the target 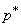 there is nothing like the probability of hitting at least one fragment in it. Using the formula (5.9.5) of chapter 5, we have:
there is nothing like the probability of hitting at least one fragment in it. Using the formula (5.9.5) of chapter 5, we have:
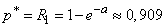 .
.
The probability of hitting a target is:
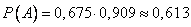 .
.
Let us use the formula (9.4.5) for the probability of hitting the circle in order to derive one important distribution for practice: the so-called Rayleigh distribution.
Consider on the plane 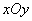 (fig. 9.4.2) random point
(fig. 9.4.2) random point 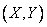 scattering around the origin
scattering around the origin 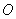 according to a circular normal law with a standard deviation
according to a circular normal law with a standard deviation  . Find the distribution law of a random variable
. Find the distribution law of a random variable 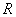 - distance from point
- distance from point 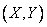 to the origin, i.e. lengths of a random vector with components
to the origin, i.e. lengths of a random vector with components 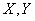 .
.

Fig. 9.4.2.
We first find the distribution function 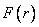 magnitudes
magnitudes 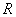 . By definition
. By definition
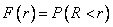 .
.
This is nothing but the probability of hitting a random point. 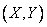 inside a circle of radius
inside a circle of radius 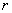 (fig. 9.4.2). By the formula (9.4.5), this probability is equal to:
(fig. 9.4.2). By the formula (9.4.5), this probability is equal to:
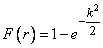 ,
,
Where  i.e.
i.e.
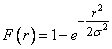 . (9.4.6)
. (9.4.6)
This expression of the distribution function makes sense only for positive values. 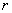 ; with negative
; with negative 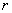 need to put
need to put 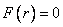 .
.
Differentiating the distribution function 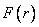 by
by 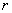 let's find the distribution density
let's find the distribution density
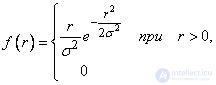 (9.4.7)
(9.4.7)
The Rayleigh law (9.4.7) is found in different areas of practice in shooting, radio engineering, electrical engineering, etc.
Function graph  (density Rayleigh law) is shown in Figure 9.4.3.
(density Rayleigh law) is shown in Figure 9.4.3.
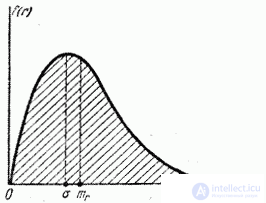
Fig. 9.4.3
Find the numerical characteristics of the value 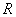 distributed according to the Rayleigh law, namely: its fashion
distributed according to the Rayleigh law, namely: its fashion 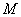 and mathematical expectation
and mathematical expectation 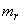 . In order to find the mode — the abscissa of the point at which the probability density is maximum, we differentiate
. In order to find the mode — the abscissa of the point at which the probability density is maximum, we differentiate  and equate the derivative to zero:
and equate the derivative to zero:
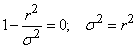 .
.
The root of this equation is the desired mode.
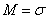 . (9.4.8)
. (9.4.8)
Thus, the most probable distance value 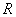 random point
random point 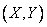 from the origin is equal to the mean square deviation of dispersion.
from the origin is equal to the mean square deviation of dispersion.
Expected value 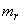 find the formula
find the formula
 .
.
Replacing a variable
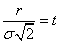 .
.
we will receive:
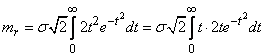 .
.
Integrating in parts, we find the expectation of the distance 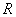 :
:
 . (9.4.9)
. (9.4.9)
Comments
To leave a comment
Probability theory. Mathematical Statistics and Stochastic Analysis
Terms: Probability theory. Mathematical Statistics and Stochastic Analysis