Lecture
The mathematical expectation is the average value of a random variable (this is the probability distribution of a random variable, considered in probability theory) [1] . In English literature it is denoted by 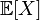 [2] (for example, from the English. Expected value or German Erwartungswert ), in Russian -
[2] (for example, from the English. Expected value or German Erwartungswert ), in Russian - 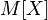 (perhaps from the English. Mean value or German. Mittelwert , and possibly from the "Mathematical expectation"). The statistics often use the designation
(perhaps from the English. Mean value or German. Mittelwert , and possibly from the "Mathematical expectation"). The statistics often use the designation 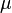 .
.
Let probabilistic space be given 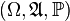 and a random variable defined on it
and a random variable defined on it 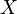 . That is, by definition,
. That is, by definition, 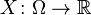 - measurable function. If there is a Lebesgue integral from
- measurable function. If there is a Lebesgue integral from 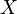 in space
in space 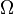 , it is called the expectation, or average (expected) value, and is denoted by
, it is called the expectation, or average (expected) value, and is denoted by 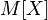 or
or 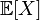 .
.

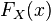 - the distribution function of a random variable, then its expectation is given by the Lebesgue – Stieltjes integral:
- the distribution function of a random variable, then its expectation is given by the Lebesgue – Stieltjes integral: 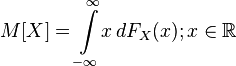 .
. 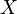 - discrete random variable with distribution
- discrete random variable with distribution 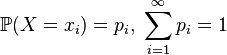 ,
, then it follows directly from the definition of the Lebesgue integral that
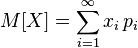 .
. 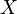 - positive integer random variable (special case discrete), having a probability distribution
- positive integer random variable (special case discrete), having a probability distribution 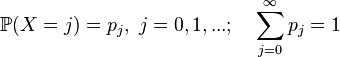
then its expectation can be expressed in terms of the generating function of the sequence 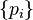
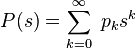
as the value of the first derivative in the unit:  . If the expectation
. If the expectation 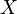 endlessly then
endlessly then 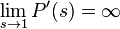 and we will write
and we will write 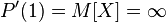
Now take the generating function  sequences of tails of distribution
sequences of tails of distribution 
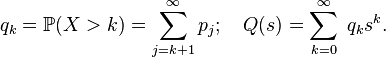
This generating function is associated with a previously defined function. 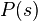 property:
property: 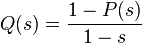 at
at  . From this, by the mean-theorem, it follows that the expectation is equal to just the value of this function in the unit:
. From this, by the mean-theorem, it follows that the expectation is equal to just the value of this function in the unit:
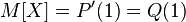
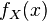 equal to
equal to 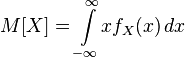 .
. Let be 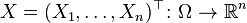 - random vector. Then by definition
- random vector. Then by definition
 ,
, that is, the expectation of a vector is determined componentwise.
Let be 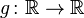 - Borel function, such that a random variable
- Borel function, such that a random variable 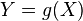 has a finite mathematical expectation. Then the formula is valid for him:
has a finite mathematical expectation. Then the formula is valid for him:
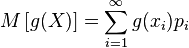 ,
, if a 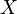 has a discrete distribution;
has a discrete distribution;
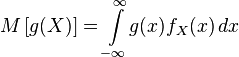 ,
, if a 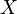 has an absolutely continuous distribution.
has an absolutely continuous distribution.
If the distribution  random variable
random variable 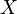 general view then
general view then
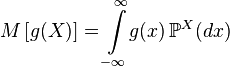 .
. In the special case when  , Expected value
, Expected value  called
called 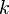 th moment of a random variable.
th moment of a random variable.

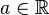 - constant;
- constant; 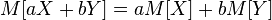 ,
, 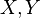 - random variables with finite mathematical expectation, and
- random variables with finite mathematical expectation, and 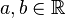 - arbitrary constants;
- arbitrary constants; 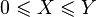 almost sure and
almost sure and  - a random variable with a finite mathematical expectation, then a mathematical expectation of a random variable
- a random variable with a finite mathematical expectation, then a mathematical expectation of a random variable 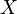 also of course
also of course 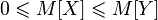 ;
; 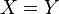 almost surely
almost surely  .
. 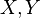 equal to the product of their mathematical expectations
equal to the product of their mathematical expectations 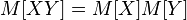 .
. 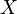 can be expressed through its moments generating function
can be expressed through its moments generating function 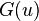 as the value of the first derivative at zero:
as the value of the first derivative at zero: 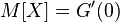
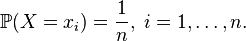 Then her expectation is
Then her expectation is 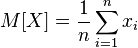
equal to the arithmetic average of all received values.
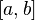 where
where 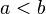 . Then its density is
. Then its density is 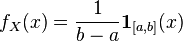 and the expectation is
and the expectation is 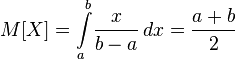 .
. 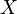 has a standard Cauchy distribution. Then
has a standard Cauchy distribution. Then 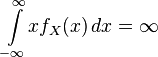 ,
, i.e. expectation 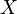 undefined.
undefined.
Comments
To leave a comment
Probability theory. Mathematical Statistics and Stochastic Analysis
Terms: Probability theory. Mathematical Statistics and Stochastic Analysis