Lecture
We have proved above that the expectation of a random variable subject to the normal law (6.1.1) is equal to 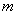 and the standard deviation is
and the standard deviation is 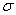 .
.
We derive general formulas for central moments of any order.
By definition:
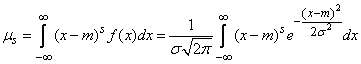 .
.
Doing a variable change
 ,
,
we will receive:
 . (6.2.1)
. (6.2.1)
Applying to the expression (6.2.1) the integration formula in parts:
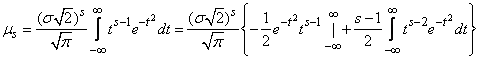 .
.
Bearing in mind that the first term inside the brackets is zero, we get:
 . (6.2.2)
. (6.2.2)
From the formula (6.2.1) we have the following expression for  :
:
 . (6.2.3)
. (6.2.3)
Comparing the right-hand sides of formulas (6.2.2) and (6.2.3), we see that they differ only by the factor  ; Consequently,
; Consequently,
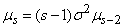 . (6.2.4)
. (6.2.4)
Formula (6.2.4) is a simple recurrence relation that allows expressing higher order moments in terms of lower order moments. Using this formula and bearing in mind that 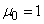 and
and 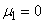 , it is possible to calculate the central moments of all orders. Because
, it is possible to calculate the central moments of all orders. Because 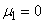 , then from formula (6.2.4) it follows that all odd moments of the normal distribution are equal to zero. This, however, directly follows from the symmetry of the normal law.
, then from formula (6.2.4) it follows that all odd moments of the normal distribution are equal to zero. This, however, directly follows from the symmetry of the normal law.
For even 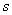 The following expressions for consecutive moments are derived from formula (6.2.4):
The following expressions for consecutive moments are derived from formula (6.2.4):
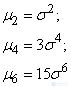
etc.
General formula for the moment 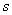 th order for any even
th order for any even 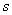 has the form:
has the form:
 ,
,
where under the symbol 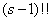 understood the product of all odd numbers from 1 to
understood the product of all odd numbers from 1 to 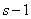 .
.
So as for normal law 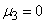 , its asymmetry is also zero:
, its asymmetry is also zero:
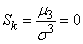 .
.
From the expression of the fourth moment
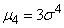
we have:
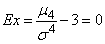 ,
,
those. the excess of normal distribution is zero. This is only natural, since the purpose of an excess is to characterize the relative steepness of this law as compared to normal.
Comments
To leave a comment
Probability theory. Mathematical Statistics and Stochastic Analysis
Terms: Probability theory. Mathematical Statistics and Stochastic Analysis