Rows of distribution. Polygon, Bar Graph, Cumulate, Ogiva

Content

1. Distribution rows
2. Polygon
3. bar chart
4. Cumulative
5. Ogiva

After determining the grouping attribute, the number of groups and grouping intervals, these reports and groups are presented in the form of distribution series and arranged in the form of statistical tables.

A number of distribution is one of the types of groupings.

Distribution series - is an ordered distribution of the units of the studied population into groups according to a certain varying characteristic.

Depending on the characteristic underlying the formation of a series of distribution, attributive and variational distribution series are distinguished:

• Attributive - called the distribution series, built on the qualitative characteristics.
• Distribution series constructed in ascending or descending order of values ​​of a quantitative trait are called variational .

The variation variation series consists of two columns:

The first column lists the quantitative values ​​of the varying attribute, which are called variants and are indicated by 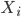. Discrete option - expressed as an integer. Interval option is between and to. Depending on the type of options, you can build a discrete or interval variation series.
The second column contains the number of specific options , expressed in terms of frequency or frequency:

Frequencies are absolute numbers that show so many times in the aggregate this characteristic value, which denote . The sum of all frequencies must be equal to the number of units of the entire population.

Frequently ( ) - These are frequencies expressed as a percentage of the total. The sum of all frequencies expressed as a percentage should be equal to 100% in fractions to a unit.

Graphic representation of distribution rows

Clearly the distribution series are represented using graphic images.

Distribution rows are depicted as:

• Polygon
• Histograms
• Cumulative
• Ogiv

Polygon

When constructing a polygon on the horizontal axis (abscissa axis), the values ​​of the varying attribute are laid, and on the vertical axis (ordinate axis), frequencies or frequencies.

Polygon in Fig. 6.1 built according to the microcensus of the Russian population in 1994

6.1. Household size distribution

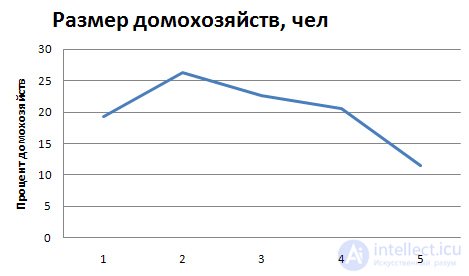
Condition : The data on the distribution of 25 employees of one of the enterprises by tariff categories are given:
four; 2; four; 6; five; 6; four; one; 3; one; 2; five; 2; 6; 3; one; 2; 3; four; five; four; 6; 2; 3; four
Task : Build a discrete variation series and depict it graphically as a distribution polygon.
Solution :
In this example, the options are the wage rate of the employee. To determine the frequencies, it is necessary to calculate the number of workers with the corresponding tariff category.

The polygon is used for discrete variation series.

To build a distribution polygon (Fig. 1), we plot the abscissa (X) along the quantitative values ​​of the varying attribute — the variants, and along the ordinate — the frequencies or frequencies.

If the attribute values ​​are expressed as intervals, then such a series is called an interval.
Interval rows of distribution are represented graphically in the form of a histogram, cumulates or ogives.

Statistical table

Condition : Data on the size of deposits of 20 individuals in one bank (thousand rubles) 60; 25; 12; ten; 68; 35; 2; 17; 51; 9; 3; 130; 24; 85; 100; 152; 6; 18; 7; 42
Task : Construct an interval variational series with equal intervals.
Solution :

1. The original population consists of 20 units (N = 20).
2. According to the Sturgess formula, we determine the required number of used groups: n = 1 + 3,322 * lg20 = 5
3. Calculate the value of the equal interval: i = (152 - 2) / 5 = 30 thousand rubles
4. We divide the initial set into 5 groups with an interval of 30 thousand rubles.
5. The results of grouping will be presented in the table:

With such a record of a continuous feature, when the same value occurs twice (as the upper limit of one interval and the lower limit of another interval), then this value belongs to the group where this value acts as an upper limit.

bar chart

To construct a histogram on the abscissa axis, indicate the values ​​of the boundaries of the intervals and on their basis build rectangles, the height of which is proportional to the frequencies (or frequencies).

In fig. 6.2. depicts a histogram of the distribution of the population of Russia in 1997 by age groups.

Fig. 6.2. The distribution of the population of Russia by age groups

Condition : The distribution of 30 employees of the company by the size of the monthly wage is given

Task : Display the interval variation series graphically in the form of a histogram and cumulates.
Solution :

1. The unknown border of the open (first) interval is determined by the magnitude of the second interval: 7000 - 5000 = 2000 rubles. With the same value, we find the lower limit of the first interval: 5000 - 2000 = 3000 rubles.
2. To construct a histogram in a rectangular coordinate system along the abscissa axis, we postpone the segments, the values ​​of which correspond to the intervals of the varietal series.
   These segments serve as the lower base, and the corresponding frequency (frequency) - the height of the formed rectangles.
3. Construct a histogram:

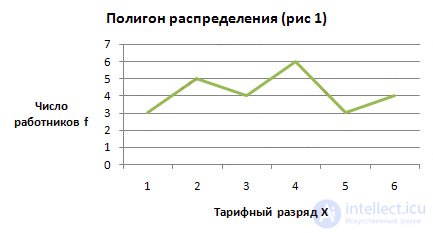
To build cumulates, it is necessary to calculate the accumulated frequencies (frequencies). They are determined by successively summing the frequencies (frequencies) of previous intervals and are denoted by S. The accumulated frequencies show how many units of the aggregate have a characteristic value not larger than the considered one.

Cumulative

The distribution of the trait in the variation series by the accumulated frequencies (frequencies) is depicted using cumulates.

Cumulative or cumulative curve in contrast to the landfill is based on the accumulated frequencies or frequencies. In this case, the characteristic values ​​are placed on the abscissa axis, and the accumulated frequencies or frequencies are placed on the ordinate axis (Fig. 6.3).

Fig. 6.3. Cumulative distribution of households by size

4. Calculate the accumulated frequency:
Knocked frequency of the first interval is calculated as follows: 0 + 4 = 4, for the second: 4 + 12 = 16; for the third: 4 + 12 + 8 = 24, etc.

When building cumulates, the cumulative frequency (frequency) of the corresponding interval is assigned to its upper boundary:

Ogiva

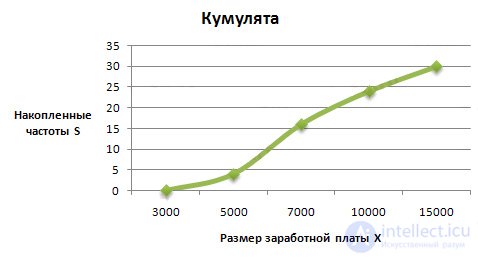
Ogiva is constructed in the same way as cumulative, with the only difference being that the accumulated frequencies are placed on the abscissa axis, and the characteristic values ​​are on the ordinate axis.

A variety of cumulates is a concentration curve or a Lorenz graph. To construct a concentration curve, a scale scale is plotted on both axes of the rectangular system in percent from 0 to 100. At the same time, the accumulated frequencies are indicated on the abscissa and the accumulated fraction (in percent) by volume of the feature are indicated on the vertical axis.

The uniform distribution of the sign corresponds to the diagonal of the square on the graph (Fig. 6.4). In case of uneven distribution, the graph is a concave curve depending on the concentration level of the trait.

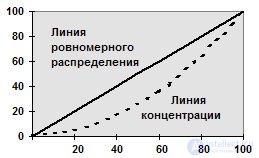
6.4. Concentration curve

See also

• Polygon and histogram