Lecture
In the previous  We considered roughly approximate methods for constructing confidence intervals for expectation and variance. In this
We considered roughly approximate methods for constructing confidence intervals for expectation and variance. In this  we will give an idea of the exact methods of solving the same problem. We emphasize that to accurately determine the confidence intervals, it is absolutely necessary to know in advance the form of the distribution law
we will give an idea of the exact methods of solving the same problem. We emphasize that to accurately determine the confidence intervals, it is absolutely necessary to know in advance the form of the distribution law  whereas it is not necessary for the application of approximate methods.
whereas it is not necessary for the application of approximate methods.
The idea of accurate methods for constructing confidence intervals is as follows. Any confidence interval is found from a condition expressing the probability of certain inequalities that include the estimate we are interested in. 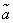 . Distribution law
. Distribution law 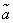 in general, depends on the unknown parameters themselves
in general, depends on the unknown parameters themselves  . However, sometimes it is possible to move in inequalities from a random variable
. However, sometimes it is possible to move in inequalities from a random variable 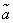 to some other function of the observed values
to some other function of the observed values 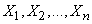 , the distribution law of which does not depend on unknown parameters, but depends only on the number of experiments
, the distribution law of which does not depend on unknown parameters, but depends only on the number of experiments 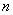 and on the type of distribution law
and on the type of distribution law  . Such random variables play a large role in mathematical statistics; they are most studied in the case of a normal distribution of magnitude
. Such random variables play a large role in mathematical statistics; they are most studied in the case of a normal distribution of magnitude  .
.
For example, it is proved that with a normal distribution of  random value
random value
 , (14.4.1)
, (14.4.1)
Where
 ,
,  ,
,
obeys the so-called student distribution law with 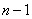 degrees of freedom; the density of this law is
degrees of freedom; the density of this law is
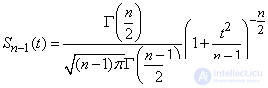 (14.4.2)
(14.4.2)
Where 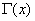 - known gamma function:
- known gamma function:
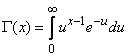 .
.
It is also proved that the random variable
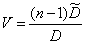 (14.4.3)
(14.4.3)
has a "distribution 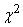 " with
" with 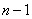 degrees of freedom (see Ch. 7. p. 145), the density of which is expressed by the formula
degrees of freedom (see Ch. 7. p. 145), the density of which is expressed by the formula
 (14.4.4)
(14.4.4)
Without dwelling on the conclusions of the distributions (14.4.2) and (14.4.4), we will show how they can be applied when building confidence intervals for the parameters 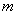 and
and 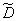 .
.
Let produced 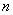 independent experiments on a random variable
independent experiments on a random variable  distributed according to normal law with unknown parameters
distributed according to normal law with unknown parameters 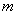 and
and 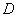 . Estimates for these parameters
. Estimates for these parameters
 ,
,  .
.
It is required to build confidence intervals for both parameters corresponding to the confidence probability. 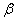 .
.
We first construct the confidence interval for the expectation. Naturally, this interval is symmetric with respect to 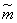 ; denote
; denote  half the length of the interval. Magnitude
half the length of the interval. Magnitude  need to choose so that the condition is met
need to choose so that the condition is met
 . (14.4.5)
. (14.4.5)
Let's try to go to the left side of equality (14.4.5) from the random variable 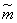 to a random value
to a random value 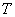 distributed by the law of student. To do this, multiply both sides of the inequality
distributed by the law of student. To do this, multiply both sides of the inequality 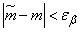 by a positive value
by a positive value 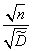 :
:

or, using the designation (14.4.1),
 . (14.4.6)
. (14.4.6)
Find such a number 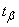 , what
, what
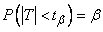 . (14.4.7)
. (14.4.7)
Magnitude 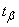 there is a condition
there is a condition
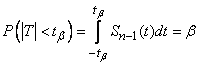 . (14.4.8)
. (14.4.8)
From the formula (14.4.2) it is clear that 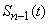 - even function; therefore (14.4.8) gives
- even function; therefore (14.4.8) gives
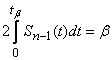 . (14.4.9)
. (14.4.9)
Equality (14.4.9) determines the value 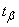 depending on the
depending on the 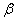 . If you have at your disposal a table of values of the integral
. If you have at your disposal a table of values of the integral
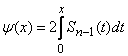 ,
,
This value can be found by reverse interpolation in this table. However, it is more convenient to make a table of values in advance.  . Such a table is given in the annex (see Table 5). This table shows the values
. Such a table is given in the annex (see Table 5). This table shows the values 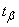 depending on confidence probability
depending on confidence probability 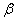 and numbers of degrees of freedom
and numbers of degrees of freedom 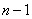 . Defining
. Defining 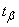 according to table 5 and believing
according to table 5 and believing
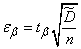 (14.4.10)
(14.4.10)
we will find half the width of the confidence interval 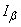 and the interval itself
and the interval itself
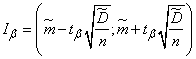 . (14.4.11)
. (14.4.11)
Example 1. Produced 5 independent experiments on a random variable  distributed normally with unknown parameters
distributed normally with unknown parameters 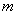 and
and 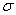 . The results of the experiments are given in table 14.4.1.
. The results of the experiments are given in table 14.4.1.
Table 14.4.1
| one | 2 | 3 | four | five |
| -2.5 | 3.4 | -2,0 | 1.0 | 2.1 |
Find a rating 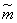 for the expectation and build for it a 90% confidence interval (i.e., the interval corresponding to the confidence probability
for the expectation and build for it a 90% confidence interval (i.e., the interval corresponding to the confidence probability 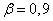 ).
).
Decision. We have
 ;
;  .
.
According to table 5 of the application for  and
and 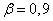 we find
we find
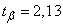 ,
,
from where
 .
.
Confidence interval will be
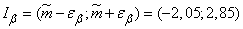 .
.
Example 2. For the conditions of example 1  14.3, assuming the value
14.3, assuming the value  distributed normally, find the exact confidence interval.
distributed normally, find the exact confidence interval.
Decision. According to table 5 of the application we find when 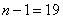 and
and 
 ; from here
; from here 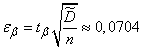 .
.
Comparing with the solution of example 1 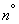 14.3 (
14.3 ( 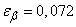 ), we are convinced that the discrepancy is very slight. If we keep the accuracy up to the second decimal place, then the confidence intervals found by the exact and approximate methods are the same:
), we are convinced that the discrepancy is very slight. If we keep the accuracy up to the second decimal place, then the confidence intervals found by the exact and approximate methods are the same:
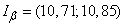 .
.
Let us proceed to the construction of a confidence interval for variance.
Consider the unbiased variance estimate.

and express the random variable 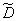 in terms of
in terms of 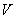 (14.4.3), having a distribution
(14.4.3), having a distribution 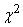 (14.4.4):
(14.4.4):
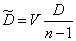 . (14.4.12)
. (14.4.12)
Knowing the law of distribution of magnitude 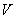 , you can find the interval
, you can find the interval 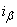 with which it falls with a given probability
with which it falls with a given probability 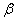 .
.
Distribution law 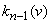 magnitudes
magnitudes 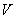 has the form shown in fig. 14.4.1.
has the form shown in fig. 14.4.1.

Fig. 14.4.1.
The question arises: how to choose the interval 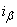 ? If the distribution law
? If the distribution law 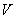 was symmetrical (like a normal law or student’s t-distribution), it would be natural to take the interval
was symmetrical (like a normal law or student’s t-distribution), it would be natural to take the interval 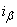 symmetric with respect to the expectation. In this case, the law
symmetric with respect to the expectation. In this case, the law 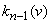 asymmetrical We agree to choose an interval so that the probability of the output value
asymmetrical We agree to choose an interval so that the probability of the output value 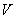 outside the interval to the right and left (the shaded areas in Fig. 14.4.1) were the same and equal
outside the interval to the right and left (the shaded areas in Fig. 14.4.1) were the same and equal
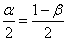 .
.
To build an interval 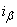 with this property, we use table 4 of the appendix: it shows the numbers
with this property, we use table 4 of the appendix: it shows the numbers 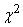 such that
such that
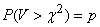
for size 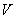 having
having 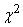 distribution with
distribution with  degrees of freedom. In our case
degrees of freedom. In our case 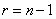 . Fix
. Fix 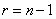 and find in the corresponding row table. 4 two values
and find in the corresponding row table. 4 two values 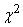 ; one that corresponds to probability
; one that corresponds to probability 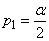 , other - probabilities
, other - probabilities 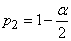 . Denote these values
. Denote these values 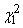 and
and  . Interval
. Interval 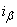 It has
It has  to your left as well
to your left as well 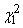 - right end.
- right end.
Now we find by interval 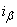 sought confidence interval
sought confidence interval 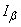 for dispersion with borders
for dispersion with borders 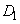 and
and 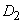 that covers the point
that covers the point 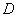 with probability
with probability 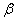 :
:
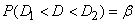 .
.
We construct such an interval 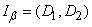 that covers a point
that covers a point 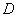 if and only if the value
if and only if the value 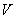 falls within the interval
falls within the interval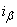 . We show that the interval
. We show that the interval
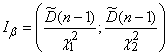 (14.4.13)
(14.4.13)
satisfies this condition. Indeed, inequalities
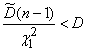 ;
; 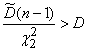
equal inequalities
 ;
; 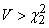 ,
,
and these inequalities are fulfilled with probability 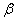 . Thus, the confidence interval for the dispersion is found and expressed by the formula (14.4.13).
. Thus, the confidence interval for the dispersion is found and expressed by the formula (14.4.13).
Example 3. Find the confidence interval for the variance under the conditions of Example 2  14.3 if it is known that the value is
14.3 if it is known that the value is  distributed normally.
distributed normally.
Decision. We have  ;
; 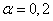 ;
; 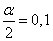 . According to table 4 of the application we find when
. According to table 4 of the application we find when 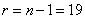
for 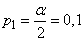
 ;
;
for 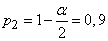
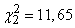 .
.
According to the formula (14.4.13) we find the confidence interval for the variance
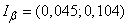 .
.
The corresponding interval for the standard deviation: 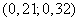 . This interval only slightly exceeds that obtained in example 2
. This interval only slightly exceeds that obtained in example 2 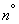 14.3 approximate spacing method
14.3 approximate spacing method 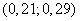 .
.
Comments
To leave a comment
Probability theory. Mathematical Statistics and Stochastic Analysis
Terms: Probability theory. Mathematical Statistics and Stochastic Analysis