Lecture
Let be 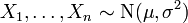 - independent sampling from the normal distribution, where
- independent sampling from the normal distribution, where  - known dispersion. Define arbitrary
- known dispersion. Define arbitrary 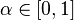 and build a confidence interval for the unknown mean
and build a confidence interval for the unknown mean 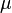 .
.
Statement. Random value
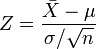
has a standard normal distribution  . Let be
. Let be 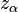 -
- 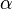 -quantile standard normal distribution. Then, due to the symmetry of the latter, we have:
-quantile standard normal distribution. Then, due to the symmetry of the latter, we have:
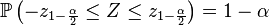 .
. After substitution of the expression for 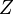 and simple algebraic transformations we get:
and simple algebraic transformations we get:
 .
. Let be 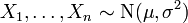 - independent sampling from the normal distribution, where
- independent sampling from the normal distribution, where 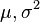 - unknown constants. Construct a confidence interval for an unknown mean
- unknown constants. Construct a confidence interval for an unknown mean 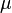 .
.
Statement. Random value
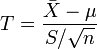 ,
, Where 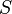 - unbiased sample standard deviation, has a Student’s distribution with
- unbiased sample standard deviation, has a Student’s distribution with  degrees of freedom
degrees of freedom 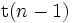 . Let be
. Let be 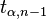 -
- 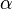 quantile student distribution. Then, due to the symmetry of the latter, we have:
quantile student distribution. Then, due to the symmetry of the latter, we have:
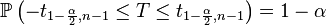 .
. After substitution of the expression for 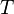 and simple algebraic transformations we get:
and simple algebraic transformations we get:
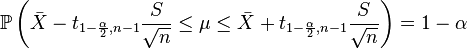 .
.
Comments
To leave a comment
Probability theory. Mathematical Statistics and Stochastic Analysis
Terms: Probability theory. Mathematical Statistics and Stochastic Analysis