18. Conjugation of circular arcs

When constructing the conjugation of circular arcs by a straight line, two problems can be considered: the conjugate straight line has an external or internal tangency. In the first task (Fig. 33, a) from the center of the arc

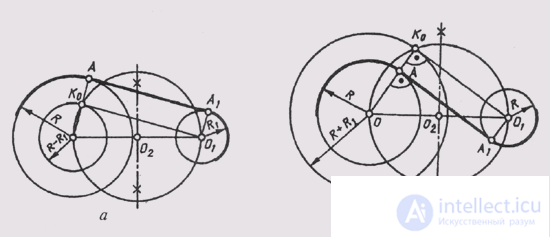
Fig. 33

the smaller radius R1 is tangent to the auxiliary circle drawn by radius R - RI. Its point of tangency, Ko, is used to build the conjugation point A on an arc of radius R.

To obtain the second interface point А _1, on the arc of radius R ₁ , an auxiliary line O ₁ A _{1 is} parallel to O A. The section of the external tangent line will be limited by points A and A ₁ .

The task of building the inner tangent line (Fig. 33b ) is solved if the auxiliary circle is built with a radius equal to R + R ₁ ,