The height, median and bisector of the triangle

Triangle height

The height of a triangle dropped from a given vertex is called a perpendicular drawn from this vertex to a straight line that contains the opposite side of the triangle.



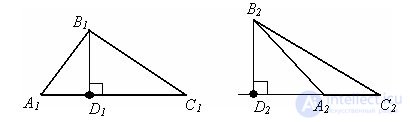
B1D1 - height of the triangle A1B1C1, dropped from the vertex B1. B2D2 - the height of the triangle A2B2C2, dropped from the vertex B2.

Triangle bisector

The bisector of a triangle drawn from a given vertex is the segment of the bisector of the angle of a triangle that connects this vertex with the point of the opposite side.



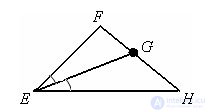
EG - FEH angle bisector. ∠ FEG = ∠ GEH.

Median of a triangle

The median of a triangle drawn from a given vertex is the segment connecting this vertex with the middle of the opposite side of the triangle.



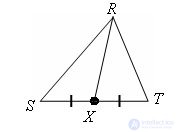
RX is the median of the SRT angle. SX = XT.