Construction of an angle equal to this

To set aside from this half-line into this half-plane an angle equal to this angle.



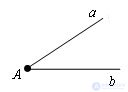
Decision.



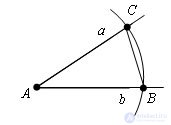
Draw a circle with an arbitrary radius and centers at the vertex A of a given angle. Let B and C be the intersection points of a circle with the sides of an angle. And spend the segment BC.



Let us draw a circle with radius AB with center at the point O - the starting point of this half-line. The intersection point of the circle with the ray is denoted by B1.



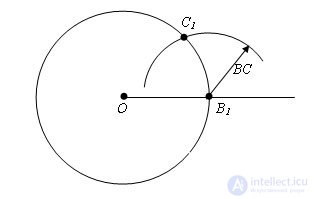
Now we describe a circle with center B1 and radius BC. Let the point C1 intersection of the constructed circles in the specified half-plane.



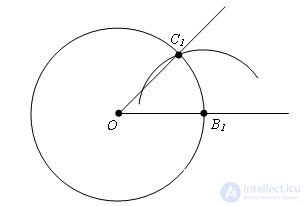
Let's draw a ray from point O, through point C1. Angle C1OB1 and will be sought.

Evidence.



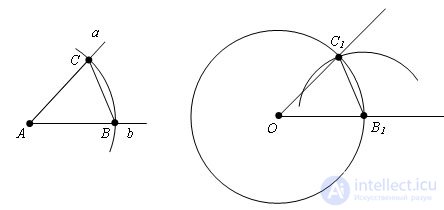
Triangles ABC and OB1C1 are equal as triangles with corresponding sides. And therefore the angles CAB and C1OB1 are equal.