The firs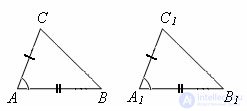t sign of equality of triangles

Theorem



If the two sides and the angle between them of one triangle are equal respectively to the two sides and the angle between them of another triangle, then such triangles are equal.

Evidence. Let triangles ABC and A1B1C1 ∠ A = ∠ A1, AB = A1B1, AC = A1C1.

Let there be a triangle A1B2C2 - a triangle equal to the triangle ABC, with vertex B2 lying on the ray A1B1, and vertex C2 in the same half-plane with respect to the straight line A1B1, where vertex C1 lies.



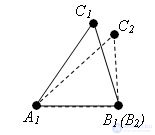
Since A1B1 = A1B2, the vertices B1 and B2 coincide.



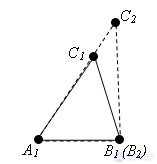
Since ∠ B1A1C1 = ∠ B2A1C2, the beam A1C1 coincides with the beam A1C2.



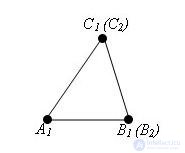
Since A1C1 = A1C2, the point C1 coincides with the point C2. Therefore, the triangle A1B1C1 coincides with the triangle A1B2C2, and therefore is equal to the triangle ABC. The theorem is proved.