Lecture
The method of converting continuous signals into a set of uncorrelated coefficients was developed by Karhunen [27] and Loeev [28]. As indicated in [30], Hotelling [29] first proposed a method for converting discrete signals into a set of uncorrelated coefficients. However, in most works on digital signal processing, both discrete and continuous transformations are called the Karhunen-Loeve transform or decomposition into eigenvectors.
In the general case, the Karhunen-Loeva transform is described by the relation
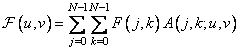 (10.8.1)
(10.8.1)
core  which satisfies the equation
which satisfies the equation
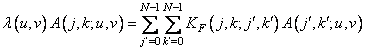 (10.8.2)
(10.8.2)
Where 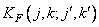 - the covariance function of the sampled image, and
- the covariance function of the sampled image, and 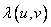 with fixed
with fixed  and
and 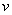 is constant. Functions
is constant. Functions  are eigenfunctions of the covariance function, and
are eigenfunctions of the covariance function, and 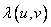 - her own values. As a rule, it is not possible to express eigenfunctions explicitly.
- her own values. As a rule, it is not possible to express eigenfunctions explicitly.
If the covariance function can be divided, i.e.
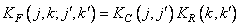 (10.8.3)
(10.8.3)
then the Karunen-Loeva decomposition core is also separable and
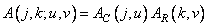 . (10.8.4)
. (10.8.4)
The rows and columns of the matrices describing these kernels satisfy the following equations:
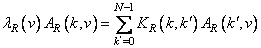 , (10.8.5)
, (10.8.5)
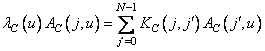 . (10.8.6)
. (10.8.6)
In the particular case when the covariance matrix describes a first-order separable Markov process, it is possible to write eigenfunctions in explicit form. For a one-dimensional Markov process with a correlation coefficient 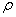 Eigenfunctions and eigenvalues have the form [3]
Eigenfunctions and eigenvalues have the form [3]
 (10.8.7)
(10.8.7)
and
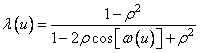 , (10.8.8)
, (10.8.8)
Where  a
a  - roots of the transcendental equation
- roots of the transcendental equation
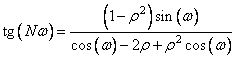 . (10.8.9)
. (10.8.9)
The eigenvectors can also be found from the recurrence formulas [32]
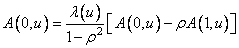 , (10.8.10a)
, (10.8.10a)
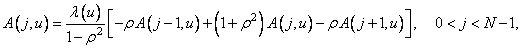 (10.8.10b)
(10.8.10b)
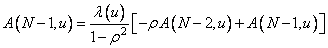 , (10.8.10b)
, (10.8.10b)
putting as initial condition 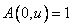 and then normalizing the resulting eigenvectors.
and then normalizing the resulting eigenvectors.
If the original and transformed images are presented in vector form, then a pair of Karhunen-Loeve transformations will have the form
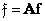 (10.8.11)
(10.8.11)
and
 . (10.8.12)
. (10.8.12)
Transformation matrix 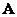 satisfies the equation
satisfies the equation
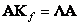 (10.8.13)
(10.8.13)
Where 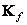 - vector covariance matrix
- vector covariance matrix 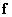 ;
; 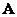 - matrix whose rows are eigenvectors of the matrix
- matrix whose rows are eigenvectors of the matrix 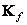 ;
; 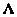 - diagonal matrix of the form
- diagonal matrix of the form
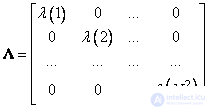 . (10.8.14)
. (10.8.14)
If the matrix 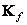 separable then
separable then
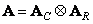 (10.8.15)
(10.8.15)
and matrix 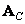 and
and 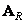 satisfy the following conditions:
satisfy the following conditions:
 (10.8.16a)
(10.8.16a)
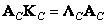 (10.8.16b)
(10.8.16b)
but 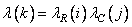 at
at 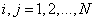 [33].
[33].
In fig. 10.8.1 shows the graphs of basis functions of the Karhunen-Loeve transform of a one-dimensional Markov process, for which the correlation coefficients of neighboring elements  .
.

Fig. 10.8.1. The basic functions of the transformation of the Karunen-Loeva with 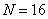 .
.
Comments
To leave a comment
Digital image processing
Terms: Digital image processing