A straight line intersecting a plane is called
perpendicular to this plane if it is perpendicular to any straight line that lies in the given plane and passes through the intersection point.
Theorem If a straight line is perpendicular to two intersecting straight lines lying in a plane, then it is perpendicular to this straight line.
 Evidence
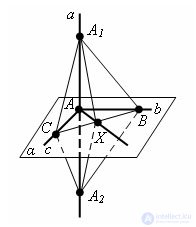
Evidence Let a be a line perpendicular to a line b and c in the plane α. Then the line a passes through the point A of the intersection of the lines b and c. We prove that the line a is perpendicular to the plane α.
Draw an arbitrary straight line x through the point A in the plane α and show that it is perpendicular to the line a. Draw an arbitrary line in the plane α that does not pass through point A and intersects lines b, c, and x. Let the intersection points be B, C, and X..
Let us put equal segments AA1 and AA2 on the line a from point A to different sides. Triangle A1CA2 is isosceles, since the segment AC is the height by the condition of the theorem and the median by construction. Triangle A1BA2 is also isosceles. Therefore, Δ A1BC = ΔA2BC on the third sign of equality of triangles.
The equality of triangles A1BC and A2BC implies the equality of angles A1BX and A2BX, therefore, the equality of triangles A1BX and A2BX by the first sign of equality of triangles. From the equality of the sides A1X and A2X, it follows that A1XA2 is isosceles. Therefore, its median XA is height. And this means that the line x is perpendicular to a. By definition, the line a is perpendicular to the plane α. The theorem is proved.

Comments
To leave a comment
Stereometry
Terms: Stereometry