Translate the current set in time form i (t) = 141 sin (ɷt + 90 °) into a complex form: exponential and algebraic.
Decision:
amplitude value of current Im = 141 A
+ 90 ° - the initial phase of the current.
We write the complex current in exponential form:
Complex current amplitude
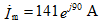
current value complex
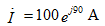
We translate into algebraic form using Euler's formula

Task 2
Algebraic voltage is specified.

Transfer to a temporary form.
Decision:
consider the stress vector on the complex plane
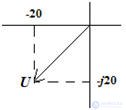
It turns out that the vector is in the third quarter of the complex plane. This means that the initial phase of the angle is from 180 ° to 270 °, i.e. add 180 ° to the resulting angle
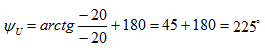
The value of the voltage module is determined by the Pythagorean theorem
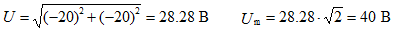
Answer: u (t) = 40 sin (ɷt + 225 °)
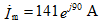
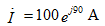



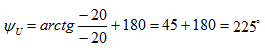
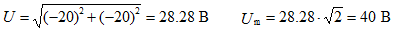
Comments
To leave a comment
Electrical Engineering, Circuit design
Terms: Electrical Engineering, Circuit design