Lecture
The kinetic energy of a body moving in an arbitrary way is equal to the sum of the kinetic energies of all n material points on which this body can be broken:
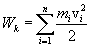
If the body rotates around a fixed axis with an angular velocity 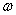 , then the linear velocity of the i-th point is
, then the linear velocity of the i-th point is 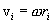 where
where 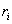 , is the distance from this point to the axis of rotation. Consequently.
, is the distance from this point to the axis of rotation. Consequently.
 | (5.11) |
Where 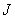 - moment of inertia of the body relative to the axis of rotation.
- moment of inertia of the body relative to the axis of rotation.
In the general case, the motion of a rigid body can be represented as the sum of two motions — a translational with a velocity equal to the velocity 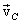 center of inertia of the body, and rotation with angular velocity
center of inertia of the body, and rotation with angular velocity 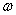 around the instantaneous axis passing through the center of inertia. The expression for the kinetic energy of the body is converted to
around the instantaneous axis passing through the center of inertia. The expression for the kinetic energy of the body is converted to
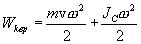 | (5.12) |
Where 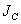 - moment of inertia of the body relative to the instantaneous axis of rotation passing through the center of inertia.
- moment of inertia of the body relative to the instantaneous axis of rotation passing through the center of inertia.
Comments
To leave a comment
Physical foundations of mechanics
Terms: Physical foundations of mechanics