Using the example of inapplicability to describe the operation of a heat engine, the laws of mechanics for a water mill demonstrate the process of the birth of a new science - thermodynamics. The development of the latter is traced in the following examples: the simplest scheme of a heat engine, the Carnot cycle, the beginnings of thermodynamics, entropy, asymmetry in nature, entropy and energy, the arrow of time, free energy, equilibrium conditions, entropy and probability, the Boltzmann formula. On this basis, a change in the scientific picture of the world in the XIX century is given, and two great theories of evolution for the living and inert worlds, Darwin and Boltzmann, are considered. Analyzed view of determinism and probability and the concept of thermal death of the universe.

I. Comparison of the heat engine and the water mill shows that the potential mechanical energy can completely transform into kinetic energy, and the potential heat energy of the heater cannot be completely transformed into mechanical work — part of the energy must remain in the refrigerator and the efficiency of the heat engine is less than 100%.

Ii. At the beginning of the 19th century, thermodynamics arose - the science of converting thermal energy into mechanical energy, and the main problem of thermodynamics was explaining why a heat engine with an efficiency close to 100% cannot be built.

In 1824, the French engineer Carnot formulated the first (the law of conservation of energy) and the second law of thermodynamics. The latter stated that the greatest efficiency of a heat engine does not depend on the working fluid and is determined only by the temperature within which the engine operates.

Iii. In 1864, the German physicist Clausius introduced the concept of entropy S = 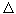Q / T where 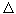Q is the heat energy consumed by the heater, T is its absolute temperature, and found that the entropy S can only increase, while the other physical parameters Q, T, P, etc., can change in any direction. The following statement was formulated: the energy in the Universe is conserved, and the entropy grows. (There is one more physical quantity that can change only in one direction - time. They say that the arrow of time and the arrow of entropy are directed in one direction.)

Iv. The Austrian physicist Boltzmann in 1896 explained this strange behavior of entropy. He established the relationship between the entropy value S and the statistical weight of the system P (the number of ways to implement this state): S = k ln P, where k = 1.38 10 ^-23 J / K is the Boltzmann constant.

V. Boltzmann showed that the most probable state of the system corresponds to a uniform distribution of particles, elements, molecules in space, i.e., the alignment of all differences. Ultimately, temperatures, pressures, velocities, etc., should level off and become uniform. This concept is called the "thermal death of the universe."

Heat engine and water mill

As already mentioned, by the beginning of the 19th century mechanics dominated physics, and various forms of motion sought to be reduced to mechanical ones. Thermal processes or transfer (movement) of heat in this world are likened to the movement of a hypothetical fluid — a thermogen.

Since ancient times, various techniques have been used to convert thermal energy into mechanical energy with the help of various heat engines. However, quite clear scientific views on this process were absent, technology was ahead of science. To understand the processes occurring in a heat engine, the analogy with a hydraulic machine was widely used.

A dam was considered, in which water fell from the height of h on the blades of the wheel and set in motion a shaft rotating at speed V. The potential energy of water mgh was converted into the kinetic energy of the shaft 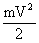. If we neglect the losses due to friction, then all potential energy passed into kinetic energy, i.e.

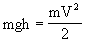
There are always two nodes in a heat engine: a heater with the temperature of the working fluid T ₁ and a refrigerator with a temperature T ₂ . Due to the flow of heat from the heater to the refrigerator, work can be performed. Here you can draw an analogy with the dam and the mill wheel and well fit the idea of ​​caloric ^* flowing from the heater to the refrigerator. However, it was noted that such an analogy does not work.

The first serious research of this process was conducted by young French engineer Sadi Carnot (1796 - 1932). He was the son of the Minister of War under Napoleon, in 1814 he fought in the suburbs of Paris with the allied forces, after the defeat of Napoleon he graduated from the Polytechnic School.

S. Carnot suffered the defeat of France in the war and sought to be useful to his homeland. He drew attention to the fact that the steam engines, which at that time were widely used in the industry of England, had a great future. Carnot believed that the country in which these machines will be the most perfect, will become a strong power. This was a stimulus for the young engineer, and he began to work on a high efficiency steam engine (efficiency, 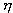). His research was published in 1824, in a small book, Reflections on the Driving Force of Fire and on Machines That Can Develop This Power.

Initially, Carnot, on the basis of the seemingly obvious analogy with a water mill, believed that, when heat is transferred from the heater to the refrigerator, the amount of heat in the heat engine is preserved. However, this was not the case.

Carnot showed that some "internal inefficiency" is inherent in the process of converting heat into work, that is, not all the amount of heat passes from the heater to the refrigerator, and some of it is necessarily lost; An expression was found for the limiting efficiency of a heat engine. You can read more about this in the Carnot Cycle paragraph. The Carnot book also contains the formulation of two principles that later received the name of the first and second principles of thermodynamics: the first start is the law of energy conservation, the second is a quantitative assessment of the above-mentioned "internal inefficiency" in converting heat into work. In the future, the last position will be considered in sufficient detail.