Comparative power radar

A new radar is being developed according to the tactical and technical requirements, where the range is set actions, accuracy of determination of coordinates and other characteristics.

Engineers have always been concerned with the question of which radar should be developed, pulsed, or continuous radiation. The answer is often determined by a given range of action and by what energy it is achieved.

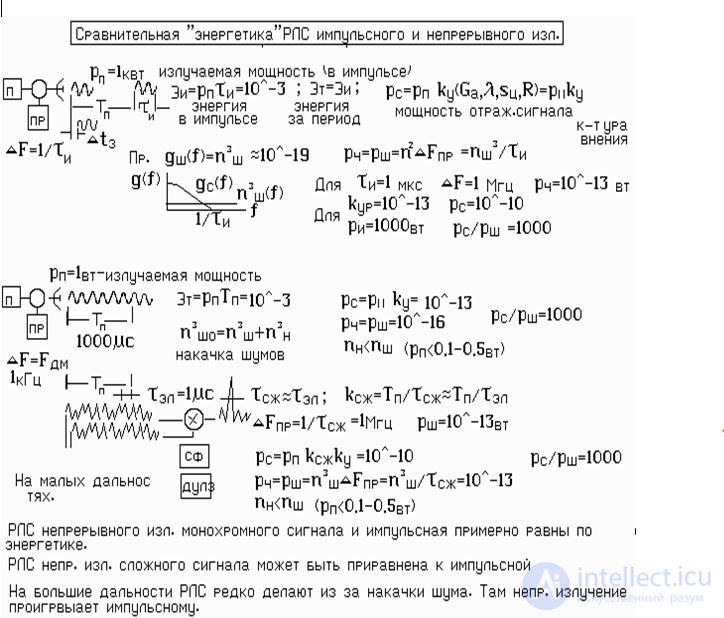
The transmitter power required to achieve a given range is determined by the radar equation, regardless of whether it is a pulsed radar or continuous radiation.

Suppose the radar antennas are the same. The radar receivers are matched with the reflected signals on the spectrum. The pulse power of the first radar is 1 kW, while the average power of a continuous radar is only 1 watt. Will the range of the pulsed radar be greater than the continuous-radiation radar?

We assume that if at the receiver output one and the same signal-to-noise ratio is obtained, then the range of action is the same.

The figure is calculated this ratio for pulsed radar. Kilovaty sounding pulse provides a 1000 times larger reflected signal than 1 watt of a continuous signal. This is true! But to receive a pulse 1 µs need a receiver in 1 MHz bandwidth, and for

radar receiver of continuous radiation of only 1 kHz (following the example of the figure). Thus , the sensitivity of the pulse receiver will be 1000 times higher than the sensitivity of the radar receiver of continuous radiation. Therefore, although the pulse reflected from the target will be 1000 times larger, the signal-to-noise ratio will be the same as in the receiver of a low-power radar of continuous radiation.

Thus, the range of the radar depends on the average transmitter power!

It is a rare case when a pulsed radar must detect a target by a single probe pulse. Then the range of different pulse radars can be compared by the energy of the probing signal.

To demonstrate the postulate of the importance of the average transmitter power, the figure shows an example of a continuous-radiation radar with FCM. The length of the code parcel FCM

1 µs The following code starts immediately after the first one.

At the receiver, the reflected signal is multiplied with the delayed transmitter code so that the maximum of the correlation function is formed. In the example, the maximum power is 1000 times greater than the reflected signal, which would have been without correlation processing.

In this way, a low-power continuous signal can be turned into a powerful pulse signal in the correlation processing receiver.

The last example confirms again that the range depends on the average power of the probing signal with a consistent processing of the reflected signals in the receiver.