Lecture
The point M o (x o , y o ) is called the maximum point of the function  , if for all points ( x, y ) belonging to a sufficiently small neighborhood of M o (x o , y o ) , the inequality
, if for all points ( x, y ) belonging to a sufficiently small neighborhood of M o (x o , y o ) , the inequality 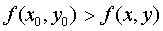 . Function value
. Function value  at the maximum point is called the maximum function.
at the maximum point is called the maximum function.
The point M o (x o , y o ) is called the minimum point of the function  , if for all points ( x, y ) belonging to a sufficiently small neighborhood of M o (x o , y o ) , the inequality
, if for all points ( x, y ) belonging to a sufficiently small neighborhood of M o (x o , y o ) , the inequality 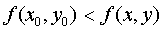 . Function value
. Function value  at the maximum point is called the minimum of the function.
at the maximum point is called the minimum of the function.
The points of maximum and minimum of a function are called the extremum points of the function, and the values of the function at these points are called the extremes of the function.
A necessary condition for the existence of an extremum FMP: if a differentiable function  reaches an extremum at point M o (x o , y o ) , then its first-order partial derivatives at this point are zero, i.e.
reaches an extremum at point M o (x o , y o ) , then its first-order partial derivatives at this point are zero, i.e. 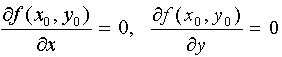 .
.
The points at which the partial derivatives are zero are called stationary points. Not every stationary point is an extremum point.
Sufficient condition for the existence of an extremum of FMF:
let M o (x o , y o ) be the stationary point of the function  .
.
Denote: 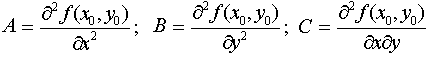 and make a ratio
and make a ratio 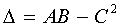 .
.
Then:
 there is an extremum, and this is the maximum if A <0 and the minimum if A > 0 ;
there is an extremum, and this is the maximum if A <0 and the minimum if A > 0 ;  Extremum is not;
Extremum is not;
Comments
To leave a comment
Mathematical analysis. Differential calculus
Terms: Mathematical analysis. Differential calculus