Antiholomorphic function

Antiholomorphic functions (also called antianalytic functions ) are a family of functions that are closely related to holomorphic functions.

Definition [edit]

Function 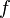defined on an open subset complex plane is called antiholomorphic if its derivative 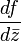by 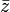exists at all points of this set. This is equivalent to the condition

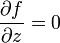
which can be given a view similar to Cauchy - Riemann conditions:

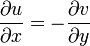
Where

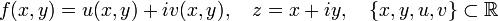
A function that depends simultaneously on and 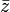, is neither holomorphic nor antiholomorphic.

Properties [edit]

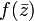• holomorphic in then and only if antiholomorphic in .
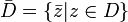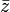• a function is antiholomorphic if and only if it can be expanded in powers in the neighborhood of each point of its domain.
• holomorphic in then and only if 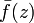antiholomorphic in .
• if a function is simultaneously holomorphic and antiholomorphic, then it is constant on any connected component of its domain.

Literature [edit]

• Shabat B.V. An Introduction to Complex Analysis. - M .: Science. - 1969, 577 pp.