- Principles of laser technology

Это окончание невероятной информации про лазер.

...

radiation acts per unit time per atom, and is equal to the inverse of the lifetime of an atom in the excited state: At „= Mtt. Einstein introduced the ATP coefficient, considering that a molecule can move from one state to another, lower energy state “without prompting from external causes”. Bohr developed this idea, saying that the system "will spontaneously transfer to a stationary state with less energy." After Bohr, the ATP coefficient was called the probability of spontaneous emission. Using the correspondence principle, Bohr related the ATP coefficient to the Functional coefficients.

dipole moment decomposition

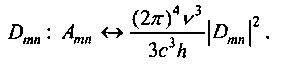
If an atom in state Ep is placed in an external electromagnetic field with frequency a), then it absorbs the field energy when this frequency coincides with the transition frequency (omn = (Ет -En) / h. As a result, the atom goes to the excited state E t Let p [a - the spectral density of the energy of electromagnetic radiation. Enter the value Wnm = Bnmpa. This value is given the meaning of the probability of absorption of radiation by the atom per unit time.

Along with the absorption process, as a result of which the transition n-> t occurs, Einstein provided the possibility of the existence of the reverse process — stimulated, stimulated, or induced emission. This process occurs when the transition t -> n under the influence of an external electromagnetic field, whose frequency is equal to the frequency of the transition. It is characterized by the value Wmn = Bmnp0J, which has the meaning of the probability of induced radiation per unit time. The coefficients of ATP, VTP, VTP are called Einstein coefficients. They are related by

The gn (or gm) effect is called the statistical weight, or the multiplicity of the degeneration of the nth (or mth) state.

Einstein's ideas about spontaneous and forced transitions associated with the emission and absorption of radiation turned out to be extremely important for the further development of the theory of radiation. These ideas became the basis for the creation of masers and lasers in our time.

Using ideas about transitions in the state of equilibrium of atoms with radiation, Einstein gave an elegant conclusion of Planck’s formula for the spectral density of radiation pv. So once again was Planck's quantum hypothesis.

However, the Einstein light quantum hypothesis, despite the successful experiments of Milliken et al., Did not inspire confidence among physicists of that time. Characterized by such an episode. When in 1913, Planck, Nernst, Rubens and Warburg put forward Einstein as a member of the Prussian Academy of Sciences, they concluded their recommendation wrote: “In general, we can say that there is hardly any of the important problems of modern physics, to which Einstein did not would make a wonderful contribution. The fact that he sometimes misses the target, as, for example, in the case of the hypothesis of light quanta, cannot be considered a negative argument, because it is impossible to put forward a new idea, even in the most accurate science, without some risk. ”

And the famous physicist Charles Barkla (Ch.Bark.la, 1877-1944), receiving the Nobel Prize in 1918 for X-ray research, said that from his experiments with X-rays it follows that radiation and absorption are continuous and that only atoms in some In exceptional cases, they emit light in quanta. Many physicists of the time believed that light quanta do not represent any physical reality, but are only a successful heuristic method for determining a certain amount of energy, possibly associated with some feature of the electromagnetic field, that is, a quantum of light was considered only as a measure and not as a kind of corpuscle. Yves 1921. The Nobel Committee formulated the award of the Einstein Prize "for his contribution to theoretical physics, and especially for the discovery of the photoelectric effect law," while not even mentioning the discovery of electromagnetic field quanta or the creation of the theory of relativity.

However, as more and more new phenomena could be explained only within the framework of quantum concepts, there was a slow and gradual recognition of the physical reality of quanta. The negative attitude of physicists to the hypothesis of light quanta is due to the fact that this hypothesis returned corpuscular ideas about light. Everyone remembered well how, after long years of discussion, the Newtonian corpuscular representations were decisively rejected, since with their help it was impossible to explain either the law of refraction of light, or interference, or diffraction. Meanwhile, light quanta can not have anything in common with Newtonian corpuscles of light. A quantum of light is a special particle propagating at the speed of light in a vacuum. The energy of a quantum of light is E = cf = hv, while along with the energy of a quantum of light, there is also an impulse equal to p-hv (c. However, the quantum of light remained a hypothetical particle until its existence was proven in the experiment.

Thus, the discovery of quanta and stimulated emitting became the starting point in the development of the theory of laser technology. The rigorous rationale for the existence of stimulated radiation and the presence of coherence was given by Dirac in 1930. Paul Adrien Maurice Dirac (fr. Paul Adrien Maurice Dirac; August 8, 1902, Bristol - October 20, 1984, Tallahassee) - English theoretical physicist, one of the creators of quantum mechanics. Winner of the Nobel Prize in Physics in 1933 (with Erwin Schrödinger). Member of the Royal Society of London (1930), as well as several world academies of sciences, including a foreign member of the Academy of Sciences of the USSR (1931), the National Academy of Sciences of the USA (1949) and the Pontifical Academy of Sciences (1961).

Dirac's works are devoted to quantum physics, the theory of elementary particles, and the general theory of relativity. He is the author of the fundamental works on quantum mechanics (general theory of transformations), quantum electrodynamics (the method of secondary quantization and the multi-time formalism) and quantum field theory (quantization of systems with constraints). The relativistic electron equation he proposed made it possible to naturally explain spin and introduce the concept of antiparticles. Other well-known Dirac results include the statistical distribution for fermions, the concept of a magnetic monopole, the hypothesis of large numbers, the Hamiltonian formulation of the theory of gravity, and others.

In September 1926, at the suggestion of Fowler, Dirac arrived in Copenhagen to spend some time at the Niels Bohr Institute. In Copenhagen, Dirac began working on the theory of radiation. In his work, Quantum Theory of Emission and Absorption of Radiation, he showed its connection with the Bose-Einstein statistics, and then, applying the quantization procedure to the wave function itself, he came to the secondary quantization method for bosons. In this approach, the state of the ensembles of the *** and particles is given by their distribution over single-particle states defined by the so-called filling numbers, which change under the action of the creation and destruction operators on the initial state. Dirac demonstrated the equivalence of two different approaches to the consideration of the electromagnetic field, based on the concept of light quanta and on the quantization of the field components. He also managed to obtain expressions for the Einstein coefficients as functions of the interaction potential and, thus, to give an interpretation of spontaneous radiation. In fact, in this work, the concept of a new physical object, a quantum field, was introduced, and the secondary quantization method formed the basis for constructing quantum electrodynamics and quantum field theory. A year later, Jordan and Eugene Wigner built a secondary quantization scheme for fermions.

Dirac continued to study radiation theory (as well as questions of the theory of dispersion and scattering) in Gottingen, where he arrived in February 1927 and where he spent the next few months. He attended Herman Weil's lectures on group theory, actively communicated with Born, Heisenberg and Robert Oppenheimer.

Works of theorists did not go unnoticed. In 1928, Rudolf Ladenburg, director of atomic physics at the Kaiser Wilhelm Society Institute of Physical Chemistry and Electrochemistry, and his student Hans Kopfermann experimentally observed population inversion (see the box "Quantum Light Amplification"), and it was in experiments with neon tubes. But the stimulated emission was very weak, and it was difficult to distinguish it against the background of spontaneous emission. Before the laser, only a step remained: in order to enhance the stimulated emission, positive feedback must be introduced into the medium, that is, placed in the resonator. But for this idea, the time has not yet come.

For the first time, experimentally induced radiation was observed by Purcell and Pound in 1950 with a non-adiabatic magnetic field in which a 7LiF crystal was placed. The Zeeman levels of the 7Li nuclei when the But field was reversed formed an inverse medium and lasing was observed in the radio frequency band at 10 MHz.

In .1939-1940, the Russian physicist V.A. Fabrikant (1907-1991) predicted the possibility of amplifying light in a plasma due to stimulated emission. In 1951, he, jointly with M. Vudynsky and F. A. Butaeva, formulated the general principle of amplification of electromagnetic radiation. They found that electromagnetic waves are amplified when passing through a medium in which the concentration of particles or their systems at the upper energy levels is excessive compared to their concentration in the equilibrium state. It was the idea of ​​a quantum amplifier.

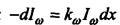To understand this idea, it is necessary to consider in more detail the process of propagation of electromagnetic radiation through matter. Let us assume that electromagnetic radiation of frequency с with density of energy flow 1a falls on a layer of matter with thickness dx. With the passage of this layer, the flow is weakened *** due to the absorption by the atoms of the substance. Reducing the energy flux density -dla proportional to the flux density of the incident radiation and the thickness of the layer

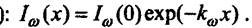The coefficient of proportionality of kyu is called the absorption coefficient. It follows that the radiation flux density at a distance x from the plane of incidence varies according to the Bouguer law (P. Bougier, 1698-1758

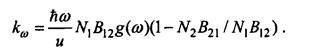Radiation absorption is resonant in nature: radiation is most absorbed at a frequency that coincides with the transition frequency between the two energy levels of the substance's aggoms. This means that the absorption coefficient has a sharp maximum at the transition frequency between the energy levels E1 and E2, with Eg> E {. If the concentration of particles at these levels is equal to N1 and N2, respectively, the absorption coefficient is determined by the formula

Here B1 and B21 are Einstein coefficients, connected by the relation g \ Bn = g2B2l, with g, (or g2) the degree of degeneration of the level Ex (or E2). The value and means the speed of radiation propagation in a given medium, g (a>) characterizes the "smearing" of energy levels.

Under normal conditions, the number of atoms in the upper energy levels is much less than their number in the lower levels, that is, N2 «N1. Indeed, at thermodynamic equilibrium, the ratio of the number of atoms at these levels is

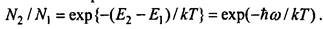For example, for visible radiation (frequency v = 7.5 • 1015 s- ') at normal temperature T = 300K, the ratio hw / kT = 1.2-103. Therefore, N2 «N1. This explains the well-known fact that, under normal conditions, radiation, passing through a substance, weakens ***, since in this case the absorption coefficient is KH> 0 ..

The question arises: can radiation pass through a medium without weakening, and, on the contrary, increase? To answer this question, we turn to the formula for the absorption coefficient. It follows from it that if the concentration of atoms with a higher energy N2 exceeds N {, then the absorption coefficient k0] becomes negative, that is, a negative absorption is said to occur. This means that the radiation is not weakened, but intensified. Thus, in order for the medium to amplify the radiation incident on it, it is necessary to provide an inverse, i.e. reversed, population of energy levels. Under the population of energy levels understand the value of Nm / gm. Inequality

g2> N] I g] is the main condition for the induced amplification. The environment in which the inverse population of energy levels is carried out is called the active medium. An important feature of the active medium is not only that it enhances the electromagnetic radiation passing through it, but at the same time, the emission spectrum narrows.

Written basic condition is necessary for the amplification of electromagnetic radiation by the active medium. However, it is not enough. The fact is that in the active medium the radiation is not only amplified, but also weakened ***. The radiation is attenuated, for example, due to absorption, due to scattering on the inhomogeneities of the medium, due to going out of its volume, etc. If the gain exceeds the total loss coefficient, then the active medium becomes an amplifier for electromagnetic radiation passing through it . For a medium to become a generator of radiation, it is necessary to use positive feedback. In this case, a part of the amplified radiation returns to the active medium and is amplified again, etc. If the gain achieved through such a connection exceeds the total losses of the amplifier and the feedback circuit, then the amplifier is self-excited and turns into a generator. To create positive feedback in the radio frequency range, use cavity resonators, and in the optical range - a system of mirrors, which are called open resonators.

The general principles of induced amplification and generation of electromagnetic radiation are implemented in modern quantum devices called masers and lasers. A maser is a quantum microwave wave amplifier. Its name - MASER is formed from the initial letters of the phrase "Microwave Amplification by Stimulated Emission of Radiation" - amplification of microwaves using stimulated emission of radiation. A laser is a quantum oscillator in the optical range. Its name - LASER is also formed from the initial letters of a similar phrase, in which the word Microwave is replaced by the word Light - light.

Since 1949, at the Physical Institute of the USSR Academy of Sciences under the leadership of Academicians of the USSR Academy of Sciences AM Prokhorov and N. G. Basov, work has been started on the study of the fine and hyperfine structure of molecular spectra by radio-spectroscopic methods. As a result of the improvement of research methods, N. G. Basov and A. M. Prokhorov in 1952 created an ammonia maser. At the same time, while developing a millimeter-wave generator at 24 00E MHz to improve the accuracy of the bombing equipment, Townes proposed the idea of ​​a new generation method - maser. Similar ideas in 1952 were put forward by Weber - the creation of the so-called “upside-down” system with respect to the Boltzmann distribution (published in 1953). In 1954, Gordon, Zeiger, and Towns published a report on the current molecular generator at NHV. The theory of this phenomenon was first developed by N. G. Bassov and A. M. Prokhorov. The creation of lasers was 5-6 years behind masers. Tauks explained this by a huge passion for masers, and A. M. Prokhorov - by the lack of proposals for the design of the resonator in the optical range and the lack of systems and methods for producing inversion. In June 1958, A. M. Prokhorov proposed using a Fabry — Perot interferometer (open resonator) as a resonator. Back in 1949, Townes and Shavlov for quantum-mechanical systems proposed using optical pumping, and the main meaning of their idea was to excite quantum particles to levels lying above the metastable state. Then, the particles along non-radiative channels accumulate at the corresponding metastable level. The three-level scheme was implemented in 1960 by Meiman and studied by fishing. At the same time, Sorokin and Stevenson proposed and implemented a four-level generation scheme for fluorite activated by uranium ions — CaF2: U3. The choice of used ions for active elements was carried out by Sorokin and Stephenson based on the fundamental works of LI Galkin and P. P. Feofilov on luminescence research. transuranium elements.

The operation of a quantum generator of any type requires the fulfillment of two resonant conditions:

Classical condition: resonance wave-resonator. The integer number of the half-waves of the generated radiation must fit within the cavity length. If L is the length of the resonator, L is the radiation wavelength, then it should be sA / 2 = L, where s is an integer.

Quantum condition: wave – atom resonance. The energy of each photon of the generated radiation must be equal to the transition energy between the two working levels of the active medium.

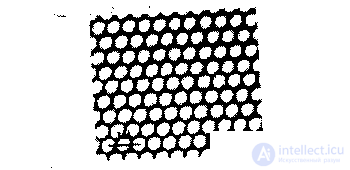
Currently developed a variety of types of lasers. Of particular importance are tunable lasers. They cover a very wide area - from the infrared range with wavelengths (2-3) - 10-3 cm to vacuum ultraviolet with wavelengths (1-2) -10-4 cm. With the help of such lasers, the possibilities of studying the properties of atoms and molecules. They allow you to detect even individual atoms. By adjusting the frequency of the laser beam, you can get into resonance with the frequency of transition between the energy levels of selected atoms. The laser power in a fraction of a watt is enough for such atoms to absorb a quantum of light and spontaneously emit a photon in an arbitrary direction. This whole process for a single atom lasts for hundredths of a microsecond. If an atom moves at a thermal velocity of a few hundred meters per second, then it intersects the laser beam with a diameter of 1 oi for several tens of microseconds. Thus, during the intersection of the laser beam, an atom has about a thousand times to absorb and emit photons. In other words, the atom glows in the laser beam. The photons emitted by atoms are characteristic of the selected atoms, even if they are in a mixture with atoms of another kind. These photons can be assembled, sent to a photomultiplier and then recorded using electronic equipment. This is one of the methods for detecting single atoms (Fig. 5.11). A more accurate and universal method of detecting atoms is based on the phenomenon of selective photoionization. In this case, the radiation is not one, but two or three lasers. The first laser “marks” the atom. For this, it is tuned to resonance with the excitation of the first level of the emitted atom. The second laser is necessary for photoionization of the “labeled” atom from the first excited state. Thus, this atom is “removed” from gas. If, when irradiated by a second laser, the atom is not ionized, and only highly excited states are reached, then a third laser is needed. As a result, the atom is converted into an ion, which is easily detected by conventional means of electronics. Thus, for example, it was possible to detect the presence of a single cesium atom in the volume where 1019 gas atoms were located (Hurst, Neyfi, Young, 1977). Modern physical devices have such fantastic sensitivity. They are used in semiconductor electronics and other areas of modern technology where an accurate assessment of impurities present in a substance is required; in controlling the state of the environment, etc. This method is particularly important in nuclear physics, where in the course of nuclear reactions at accelerators or other conditions, a small number of new atoms formed are investigated. The atomic “nanoscope” created with the help of a laser allows one to study individual atoms (A. Steane, Nature, 414, p. 24, 2001). Thus, it is now possible to directly see that for two and a half millennia it was considered fundamentally inaccessible not only for observation, but also for knowledge! In addition to the above-mentioned laser methods for detecting atoms, there are also methods for studying atomic structures using such facilities as an electron microscope, a scanning tunneling microscope, etc. As an example, the figure shows a photograph of gold atoms taken with an electron microscope.

CO2 In 1961, Helverts first proposed a Q-factor modulation method, which made it possible to significantly increase the radiation power by reducing the

pulse duration up to 10 ~ 8 ... 10 ~ 9 s.

Thus, the development of quantum electronics went from the radio band to the optical band. This is natural and due to a number of circumstances. In the field of radio spectroscopy, intensive work was carried out on the creation of powerful highly stable coherent generators, while in optics they were not interested in the stability problem at all, and they did not even think about creating quantum generators of the optical range. In optics, in contrast to radio spectroscopy, they used only sources of spontaneous radiation. The properties of active bodies and resonators have a decisive influence on the characteristics of the radiation. This aspect of quantum electronics was widely and comprehensively studied, in particular, by scientists of Ukraine, where research began in 1961. The dispersion resonator created in 1963 by V.L. Brode, M.S. Soskin and N.F. Prokopiuk laid the foundation for a new direction in quantum electronics - tunable lasers, on the basis of which, under the direction of M. S. Soskin, fundamental studies of the physical features of generation in condensed media were carried out. On the basis of a tunable laser in 1967, V.I. Kravchenko and M.S. Soskin created a new class of tunable lasers, sweep lasers, on the basis of which the high-resolution, high-resolution gapless spectroscopy method was developed and a complex of unique laser spectrometers was created. A large complex of studies was carried out under the guidance of Acad. Academy of Sciences of the USSR M. Brodin on the creation of A966) and the study of semiconductor lasers with two-photon laser pumping. Multifaceted studies on the mode of operation of a Q-switched laser were performed under the guidance of Acad. Academy of Sciences of the USSR M.P. Foxes. The creation of lasers on a wide class of organic dyes and the study of the features of their generation was carried out under the guidance of Acad. Academy of Sciences of the USSR A. Ya. Usikov at the Institute of Radio Electronics of the Academy of Sciences of the Ukrainian SSR and Corr. M. T. Shpak at the Institute of Physics, Academy of Sciences of the Ukrainian SSR. The works of Ukrainian physicists in developing the physical fundamentals of controlling the frequency of stimulated radiation and creating tunable lasers were awarded the State Prize of the Ukrainian SSR in science and technology for

1974. Thanks to many years of fundamental research in the field of quantum electronics in the USSR (A.M. Pro-Prokhorov, N.G. Basov) and in the USA (Towns, Shavlov), in the early 1960s, the first millimeter-quantum quantum generators were created (masers) and optical (lasers) ranges. Apparently, the year of birth of quantum electronics should be considered as 1954, when generation in the centimeter range was first obtained. For the development of a new principle of generation and amplification of electromagnetic waves based on molecular generators in 1959, N. G. Basov and A. M. Prokhorov were awarded the Lenin Prize. In 1964, N. G. Basov, A. M. Prokhorov, and C. Towns were awarded the Nobel Prize in Physics for basic research in the field of quantum electronics. In the same year, for the creation and study of semiconductor lasers, the Lenin Prize was awarded to the employees of the Physics Institute of the USSR Academy of Sciences B. M. Vulu, O. N. Krokhinu, Yu. M. Popov, A. P. Shotov,

D. N. Nasledov, S. M. Ryvkin, A. A. Rogachev, B. V. Tsarenkov. Prospects for the development of quantum electronics led to an avalanche-like increase in information on the study and implementation of the properties of quantum generators: only in 1956, more than 2000 articles were published on quantum generators of the optical range, and over a decade (I960 .... 1969) in this area extensive theoretical and experimental studies

In 1969, under laboratory conditions at the Physical Institute of the USSR Academy of Sciences, a CO2-N2-He mixture obtained a radiation power in a continuous mode of 8 kW (laser length 185 m, generation wavelength 10.6 μm). (Note that a 150 ... 200 W nACO2 laser burns a hole in a brick in a few minutes in a continuous mode). One of the specific features of such lasers is extremely high efficiency, which can be increased to 40%. This is due to the high efficiency of excitation of nitrogen molecules, the presence of resonant energy transfer from excited nitrogen molecules to the carbon dioxide molecule, which has a long lifetime (about 0.01 s) of the metastable level 00 ° 1. The life span of industrial model gas tubes is up to 500 ... 2000 h for He — Ne lasers, 500 ... 1000 h for ion lasers, 100 ... 2000 h for molecular lasers in the USA. In 1972, a laser based on igthrieumo-aluminum garnet (YAG: Nd3 +) crystals was demonstrated, which generated a power of 1.5 W in a continuous mode during solar pumping. A record value of efficiency — 30% for a verdotel laser was obtained in 1966 at the YAG: No3 + at 77 K. For clarity, we compare the output power of the laser with the solar optical parameters. In the visible range, the surface of the Sun radiates energy almost like an absolutely black body with a temperature of 6000 ° C. The power of solar radiation in the entire wavelength range of about 7 kW with 1 cm2 surface. At first glance, it seems that a power of 7 kW with 1 cm2 is a lot, but in reality this is far from the case, since solar radiation covers a huge range of wavelengths. For example, in a band of 1 kHz at a wavelength of X = 488 nm (one of the wavelengths generated by an Ar-laser), the radiation power is 10 ~ 5 W, i.e., to get half a power of 1 W, it is necessary to collect radiation from 10 m2 of solar surface. At the same time, using an argon – argon laser at the same wavelength, today we obtain a power of about 0.5 ± 1 kW with a similar half width and a beam divergence of less than 1 °. Thus, the output power of commercial 1-kHz gas lasers has a radiation bandwidth of approximately 108 times the power emitted by 1 cm2 of the solar surface. Using semiconductor lasers, a record inertia-free system was obtained. Thus, the laser switching time of gallium arsenide (GaAs) reached a switching time of 10 0, which made it possible to create light pulse generators with a frequency of 1010 Hz (A0 GHz) and a duration of 10 ~ 10 s. The energy required for transmitting the binary code of information on lasers is of the order of 10 ^ 16 VT> while in the microwave range and non-coherent system, the order of 10 ~ 7 ... 10 ~ 8 W is required. One of the most unique features of gas laser devices is their frequency stability, on which modern frequency standards are based. On the basis of lasers, it was possible to reduce the measurement error of the speed of light from 100 to 0.5 m / s, which made it possible to create a single standard for measuring time and length. Thanks to the frequency standards of microwave coles ***, mankind for the first time carried out, independently of astronomical observations, a precise time measurement based on molecular constants. It became possible to measure time intervals with an accuracy of twelve signs, which corresponds to the measurement of a length of time, for example, in 100 thousand years (A05 years) with an accuracy of fractions of a second. The first quantum clock was created in 1957 on the basis of an ammonia laser. At present, based on the Cesium-133 hyperfine structure line (F = 4 -> - F = 3) 9192, 631830 MHz with a half width of 200 Hz, an atomic chronometer (atomchron) is created, whose frequency stability is 10 "n, i.e. The deviation from the normal course of the clock is of the order of 0.1 s over 300 years. Even more accurate atronchrometers are made on atomic hydrogen. The best standard of the microwave frequency is a hydrogen maser that generates at a frequency (F = 1 - + - F = 0) 1.4 GHz. The accuracy of measuring time intervals is limited by the uncertainty relation Av A / ^ 1. In the optical range, where the frequencies 1014 ... 1015 s, it is possible to improve the accuracy of measuring time intervals up to the 13th sign, because it is possible to determine the resonance with an accuracy of up to 10 ~ 5-10 ~ 4 from the line width in the microwave range (Av / v ^ 10 ~ 8 .... 10 ~ 9), and in optical it can be done two to three orders of magnitude higher, that is, Av / v ^ 10 ~ 10 ... ... ju-13. Atomic chronometers are indispensable in space navigation. With the help of an atomic chronometer, a weak unevenness of the Earth's rotation was detected (at the end of October, the rotation slows down by 0.53 s, and at the end of May it accelerates by 0.065 s). Recent years have been marked by colossal advances in laser technology (M. Perry, G Mourou, Science, 264, p. 917-924 (Shau 13, 1994)). A laser with a record peak power of 1015 watts was created in the laboratory of Lurex in Livermore (USA). Heavy-duty lasers are also being created in other laboratories. When focusing radiation, energy flux densities from 1018 to 1023 W / cm2 are achieved. The energy of such radiation is concentrated in very short pulses with a duration of about several pico-, femto- and even atgo-seconds (1 a = 10-18 s). It is expected that fantastic intensities will soon be reached at the level of 1026 -1028 W / cm 2 (G. A. Mourou, S. PJ Barty, M. D. Perry, Phys. Today, 51 (1), p. 22, 1998; I Kapteyn, M. Murnane, Phys. World, 12 (1), p. 33, 1999). In this case, lasers are created not only in the optical range, but also in the X-ray — gaps and gamma-range — grazers.

With an enormous power of laser radiation, the production of an electron-positron pair can occur. Estimation of the power required for this can be carried out according to Schwinger (J. Schwinger, Phys. Rev. 82, p. 664, 1951). The generated particles must have an energy of the order of m0c2 during their lifetime of the order of r «Ω / t0c2. The corresponding characteristic length of st is the Compton length Xc = Ы ш0с. At this length, the radiation field must supply the particles with the necessary energy. Thus, the Schwinger field Es = m0c2 / eXc is of the order of Es x 2-U16B / cl {. This field corresponds to the intensity of about / L. =

= 1030 W / cm 2. At radiation intensities much lower than the specified value, the probability of pair production can be considered low.

Calculations show that in the field of high-power laser radiation, charged particles can be accelerated to colossal energies of the order of Tera eV and Peta eV. So, perhaps, in the near future, in nuclear physics, instead of expensive, traditional charged particle accelerators, which are enormous in size - these are entire factories, compact mobile laser accelerators will be used. They can be used to carry out nuclear reactions. Already, there are experiments that demonstrate the splitting of atomic nuclei in a high-power laser field, including uranium fission (RWD Ledingham et al. Phys. Rev. Lett. 84, p. 899, 2000; T. E. Cowan et al. Phys. Rev Lett. 84, p. 903, 2000). High-power lasers are also intended to be used to solve the problem of controlled thermonuclear fusion. There is even a special program in this direction — laser synthesis (O. N. Krokhin, UFN, 172 (12), p. 1466, 2002). Recent successful experiments (S. Fritzler et al. Phys. Rev. Lett. 89, p. 165004, 2002) inspire hope that this program may well be implemented.

Quantum electromagnetic radiation generators are created by scientists in physical laboratories. However, it turned out that masers exist in nature, in particular, in interstellar space. The existence of masers in interstellar space is due to the fact that there are atoms and even complex molecules in this space. The first interstellar molecule was discovered in 1937. It was a free chemical radical CH. However, hydrogen is the most common. This was proven in 1951, when radiation with a wavelength of 21 cm was recorded, corresponding to a transition between two very similar states of the hydrogen atom. In 1963, radio astronomers discovered hydroxyl - OH in the interstellar medium. Then more complex molecules were discovered, in particular, ammonia molecules and water vapor. Studies have shown that hydroxyl radiation comes from areas where temperatures are higher than in the interstellar medium. In this case, most often in the radio spectrum of these regions, hydroxyl did not give an absorption line, but a rather narrow intense emission line. This meant that OH molecules are more “hot” than their environment. In fact, the emission of a molecule is possible only if it is in an excited state. But, having emitted a photon, it loses energy, and in order for the radiation to again occur, somehow the molecule must again go into an excited state. This means that a giant maser “works” in such areas, in which the inverse population of hydroxyl energy levels is constantly created. In the same places where powerful hydroxyl maser radiation comes from, intense lines of water vapor emission are also observed. So it also works on a water vapor maser. In the areas of work of masers, the concentration of particles reaches 108 cm ~ b, and the temperature varies from several hundred to 1000 K. Conditions for the emergence of maser exist in the so-called protostar and in the outer areas of some very cold stars (D. Rank, C. Townes, U. Welch, UFN, 112 (2), 1974). Cosmic masers are found on more than 100 molecules (C. X. Townes, Quantum Electronics, 24, p. 1063, 1997) and on excited hydrogen atoms (V. Strelnitski et al. Science, 272, p. 1459, 1996). In the atmospheres of Mars and Venus, stimulated emission of a CO2 molecule in the infrared region of the spectrum was noted (M.J. Mumma et al. Science, 212, p. 45, 1981). Along with the maser effect, there is also a cosmic laser effect (V.S. Jlemoxoe, UFN, 172 (12), p. 1468, 2002).

Продолжение:

Часть 1 Principles of laser technology
Часть 2 - Principles of laser technology