3.1.2. Integral table. Definition and formulas

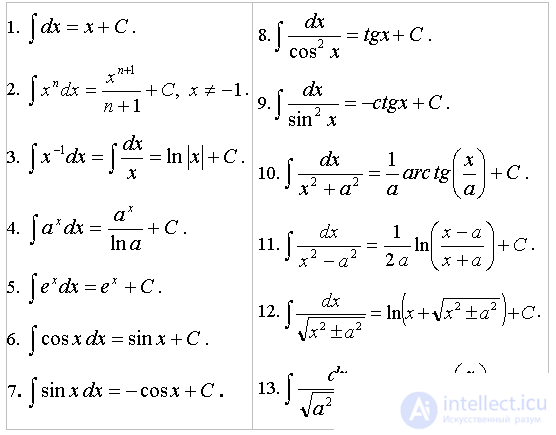
Integration is one of two basic operations in mathematical analysis. Unlike the differentiation operation, the integral of an elementary function may not be an elementary function. For example, it follows from the Liouville theorem that the integral of not an elementary function. Tables of known primitives are often very useful, although now they lose their relevance with the advent of computer algebra systems. This page lists the most common primitives.

used as an arbitrary integration constant that can be determined if the value of the integral is known at some point. Each function has an infinite number of antiderivatives.

Content

• 1Rules for integrating functions
• 2 Integrals of elementary functions
  • 2.1Rational functions
  • 2.2Logarithms
  • 2.3Exponential Functions
  • 2.4Rational functions
  • 2.5Trigonometric functions
  • 2.6 Hyperbolic functions

Function Integration Rules

Integrals of elementary functions

Rational functions

List of integrals of rational functions

(antiderivative from zero is a constant, in any limits of integration the integral from zero is zero)

}

Evidence

Make a replacement get

("High logarithm")

Logarithms

List of integrals of logarithmic functions

Exponential Functions

List of integrals of exponential functions

Irrational functions

List of integrals of irrational functions

Differential bean

("Long logarithm")

Evidence

Let be , suppose also that . Let's use hyperbolic functions, we will make replacement

But

therefore

From here, including the logarithm of the denominator of the last fraction in the constant C, we get

If a then replacing we reduce the integral to the case already considered. If , then we make a replacement and carry out arguments similar to the case considered [1].

Trigonometric functions

list of integrals of trigonometric functions , list of integrals of inverse trigonometric functions

Evidence

Evidence

Hyperbolic functions

List of integrals of hyperbolic functions

also

also

Proof of

Proof of formula :

Proof of formula : .

Proof of formula :