3.1.1. Definition of indefinite integral

The function F ( x ) is called primitive of the function f ( x ) on the interval [a; b] if the equality F ' ( x ) = f ( x ) holds at all points of this segment.

If F ( x ) is the antiderivative of the function f ( x ) , then F ( x ) + C is also antiderivative of this function. The set of all primitives F ( x ) + C of the function f ( x ) is called the indefinite integral of the function f ( x ) and is denoted by .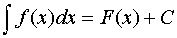

Symbol 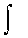is called an integral , f ( x ) is called an integrand , f ( x ) dx is called an integrand , x is called an integration variable .

The indefinite integral has the following properties.