Lecture
Let the domain D be regular in polar coordinates, i.e. the line φ = c , ( c - const ) intersects the boundary of the domain D no more than two times. Let the domain D be given by the inequalities β ≤ φ ≤ α , ρ 1 ( φ ) ≤ ρ ≤ ρ 2 ( φ ).
Then the double integral 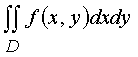 the functions f ( x, y ) , given in rectangular coordinates, can be reduced to calculating the double integral in polar coordinates:
the functions f ( x, y ) , given in rectangular coordinates, can be reduced to calculating the double integral in polar coordinates:
.
Comments
To leave a comment
Mathematical analysis. Integral calculus
Terms: Mathematical analysis. Integral calculus