7 . Separable topological spaces

Determination 16. If topological n a space of X and s an n th b olee h eat countable n odmnozhestvo A , of amykanie to otorrhea with necessarily represent with X , ie about it -called tsya separable . Otherwise, the space is said to be inseparable .

For a metric space (X, d ) this means that there is a sequence x 1 , x 2 , ... of elements from X such that for " x Î X, " e > 0 $ n ( e , x): d (x n , x) < e .

Example 20 . s is a separable space. Indeed, consider j r a subset of s sequences whose coordinates are rational numbers and, starting from some number, all coordinates are equal to 0. If we denote j r ( n ) - the set of sequences in which the first n coordinates are rational numbers, and the subsequent coordinates are 0, then this set will be countable, as a finite union of countable sets. Since j r = j r ( n ), it is countable, as a countable union of countable sets. On the other hand, for a predetermined e > 0 there is a number m such that

...

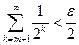Then for any sequence х = { x n } and any n there is a rational number r n such that | r n - x n | < e / 2. We denote by r a sequence in which the first m coordinates are equal to r n and the subsequent ones are equal to 0. Then r Î j r and

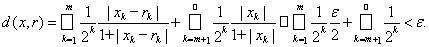
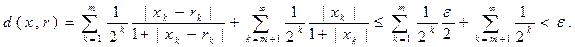
Example 21 . l p , 1 £ p < ¥ - separable. In this case, the same set j r from the previous example can be taken as a countable everywhere dense subset . In this case, rational numbers are selected based on the inequalities: | r n - x n | < e / 2 n / p (check the required inequality yourself).

Example 22 . m - inseparable. To verify this, we prove the following statement.

Lemma 1. If in a metric space X $ d > 0 and an uncountable set {x a }: d (x a , x b ) ³ d , a ¹ b , X is an inseparable space.

Proof . On the contrary, assume that X is separable. Then there exists a countable set {y k } such that for e = d / 2, S e (y k ) = X. Since {y k } is countable, {x a } -uncountable, then there is at least one ball S e (y k ), which contains more than one element from {x a }. Let x a , x b Î S e (y k ) a ¹ b . Then d £ d (x a , x b ) £ d (x a , y k ) + d (y k , x b ) <2 e = d and d < d - a contradiction.

To prove that the space m is inseparable, it suffices to use this lemma. Elements from m with coordinates equal to either 0 or 1. Then the distance between different elements of this family is equal to one as the required family . Using Cantor's diagonal method, one can make sure that the considered family is uncountable.

We point out without proof that the separability of a topological space implies the presence of a countable base in it. The converse is generally not true. However, in the case of metric spaces, the presence of a countable base of the topology implies separability.