Examples of solving problems to the section differential equations

Example N 1

Find the general solution of the equation .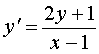

Decision.

Because 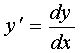, the equation has the form: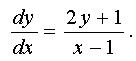
Multiplying the entire equation by dx , we get:
Dividing the whole equation by 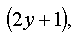we arrive at an equation with separable variables: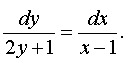

Integrating, we get:

Answer: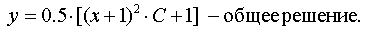

Example N 2

Find the general solution of the equation .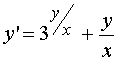

Decision.

The equation is homogeneous, since the function on the right-hand side is homogeneous:

.

Make the substitution: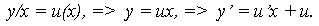

Transform the original equation:

Got an equation with separable variables: 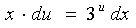. Divide the equation by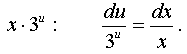

Integrating by term, we get:

Answer: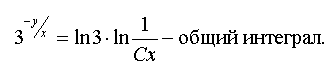

Example N 3

Find the general solution of the equation y ' + 3 x ² y + x ² = 0.

Decision.

The equation is linear with respect to the function y and its derivative y ' . We look for a solution in the form y = u · v , where u and v √ are functions of x . Because 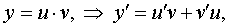then the original equation takes the form: or .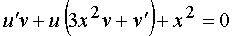

The function v is found from the equation 3 x ² v + v ' = 0, while the original equation is simplified: u'v + x ² = 0. Find the function v :

The function is found from the equation u'v + x ² = 0 with :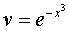

Answer:

Example N 4

Solve the Cauchy problem: y "+ y = x , y (0) = 1, y ' (0) = 1.

Decision.

1. Find y ₀ . To do this, we first find the roots of the characteristic equation k ² + 1 = 0, k = ± i . Therefore, the general solution of a homogeneous equation has the form: .
   
   
2. Find y _* . Since the right side of the equation is f ( x ) = x ( α = 0 is not the root of the characteristic equation, the degree of the polynomial is equal to one), we look for the particular solution y _* in the form: y _* = ( Ax + B ) . Find y _* ^' = A , y _* ^" = 0. Substituting y _* , y _* ^' , y _* ^" into the original equation, we get Ax + B = x . This equation 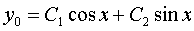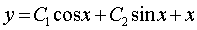is satisfied when A = 1, B = 0. Therefore, y _* = x . In this way, - common decision.

1. We define С ₁ and С ₂ so that the initial conditions are fulfilled:

   
   

Substituting the found constants С ₁ and С ₂ into a general solution, we obtain the solution of the Cauchy problem: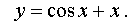

Answer: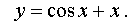

Example N 5

Find the general solution of the differential equation: .

Decision.

The general solution of the original equation is equal to the sum of the general solution of the homogeneous equation y ₀ and the particular solution y _* .

1. Find y ₀ . Find the roots of the characteristic equation:

   

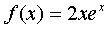2. Find y _* . Since the right side of the equation , ( α = 1 is not the root of the characteristic equation, the degree of the polynomial is equal to one), then we look for the particular solution y _* in the form: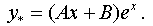
   Find

   

   Substituting y _* , y _* ^' , y _* ^" into the original equation, we get:

   

   Dividing by e ^x , after bringing similar ones we get:

   

   Comparing the coefficients at the same degrees, we compose a system of linear algebraic equations:

   

   finding a solution to this system:

   

   write the general solution:

   

Answer: