Convenient, fast, simple and quite functional Paint - in order to draw online and share with friends
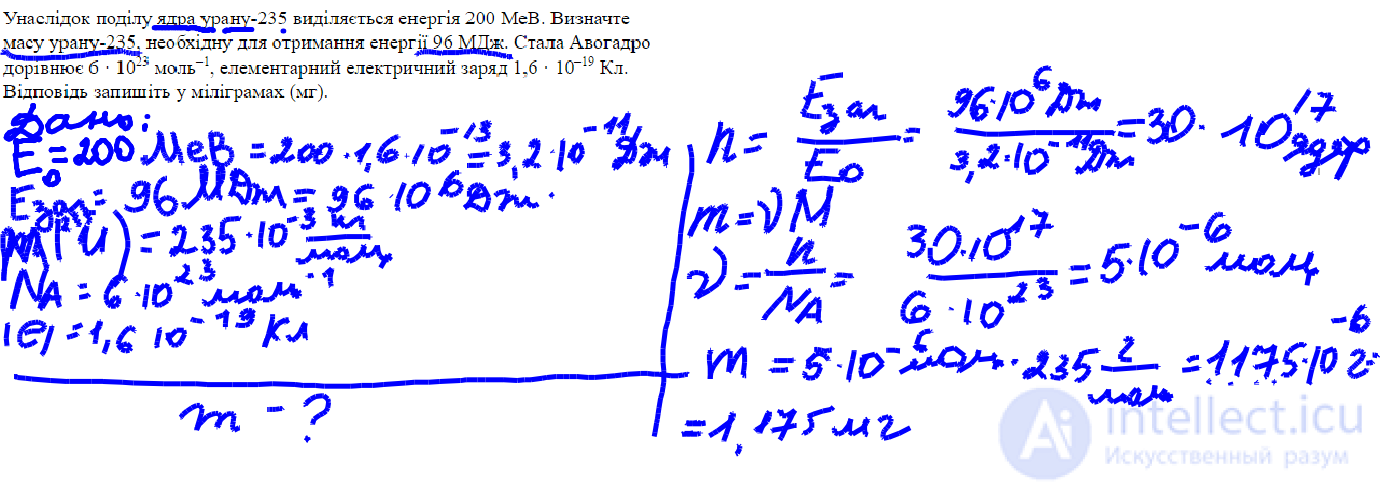
The fission of a uranium-235 nucleus releases 200 MeV of energy. Determine the mass of uranium-235 required to produce 96 MJ of energy. Avogadro's constant is 6 • 10²³ mol⁻¹, the elementary electric charge is 1.6 • 10⁻¹⁹ C. Write the answer in milligrams (mg).
Given:
The fission of one uranium-235 nucleus releases energy:
E₁ = 200 MeV = 200 × 10⁶ eV
We need to determine the mass of uranium-235 required to obtain the energy:
E = 96 MJ = 96 × 10⁶ J
Avogadro constant:
Nₐ = 6 × 10²³ 1/mol
Elementary charge:
e = 1.6 × 10⁻¹⁹ C (given, but not needed for the solution)
1. Convert the fission energy of the nucleus to joules:
1 eV = 1.6 × 10⁻¹⁹ J
So:
𝐸1=200×10^6 × 1.6×10 ^−19 =3.2×10^−11 J
2. How many nuclei are needed to obtain 96 MJ:
𝑛=96×10^6 /(3.2×10^−11) = 3 × 10^18 nuclei
3. How many moles of uranium is this:
𝜈 =3×10^18 / 6 ×10^23 = 5×10^−6 mol
4. Let's find the mass by the amount of substance:
Molar mass of uranium-235: M = 235 g/mol
𝑚 =𝜈⋅𝑀=5×10^−6 ⋅ 235 = 1.175×10−3 g= 1.175 mg
Answer:
1.175 mg